[/caption]
Atoms are made of protons, neutrons and electrons. If you cram them together and heat them up you get plasma where the electrons are only loosely associated with individual nuclei and you get a dynamic, light-emitting mix of positively charged ions and negatively charged electrons. If you cram that matter together even further, you drive electrons to merge with protons and you are left with a collection of neutrons – like in a neutron star. So, what if you keep cramming that collection of neutrons together into an even higher density? Well, eventually you get a black hole – but before that (at least hypothetically) you get a strange star.
The theory has it that compressing neutrons can eventually overcome the strong interaction, breaking down a neutron into its constituent quarks, giving a roughly equal mix of up, down and strange quarks – allowing these particles to be crammed even closer together in a smaller volume. By convention, this is called strange matter. It has been suggested that very massive neutron stars may have strange matter in their compressed cores.
However, some say that strange matter has a more fundamentally stable configuration than other matter. So, once a star’s core becomes strange, contact between it and baryonic (i.e. protons and neutrons) matter might drive the baryonic matter to adopt the strange (but more stable) matter configuration. This is the sort of thinking behind why the Large Hadron Collider might have destroyed the Earth by producing strangelets, which then produce a Kurt Vonnegut Ice-9 scenario. However, since the LHC hasn’t done any such thing, it’s reasonable to think that strange stars probably don’t form this way either.
More likely a ‘naked’ strange star, with strange matter extending from its core to its surface, might evolve naturally under its own self gravity. Once a neutron star’s core becomes strange matter, it should contract inwards leaving behind volume for an outer layer to be pulled inwards into a smaller radius and a higher density, at which point that outer layer might also become strange… and so on. Just as it seems implausible to have a star whose core is so dense that it’s essentially a black hole, but still with a star-like crust – so it may be that when a neutron star develops a strange core it inevitably becomes strange throughout.
Anyhow, if they exist at all, strange stars should have some tell tale characteristics. We know that neutron stars tend to lie in the range of 1.4 to 2 solar masses – and that any star with a neutron star’s density that’s over 10 solar masses has to become a black hole. That leaves a bit of a gap – although there is evidence of stellar black holes down to only 3 solar masses, so the gap for strange stars to form may only be in that 2 to 3 solar masses range.
The likely electrodynamic properties of strange stars are also of interest (see below). It is likely that electrons will be displaced towards the surface – leaving the body of the star with a nett positive charge surrounded by an atmosphere of negatively charged electrons. Presuming a degree of differential rotation between the star and its electron atmosphere, such a structure would generate a magnetic field of the magnitude that can be observed in a number of candidate stars.
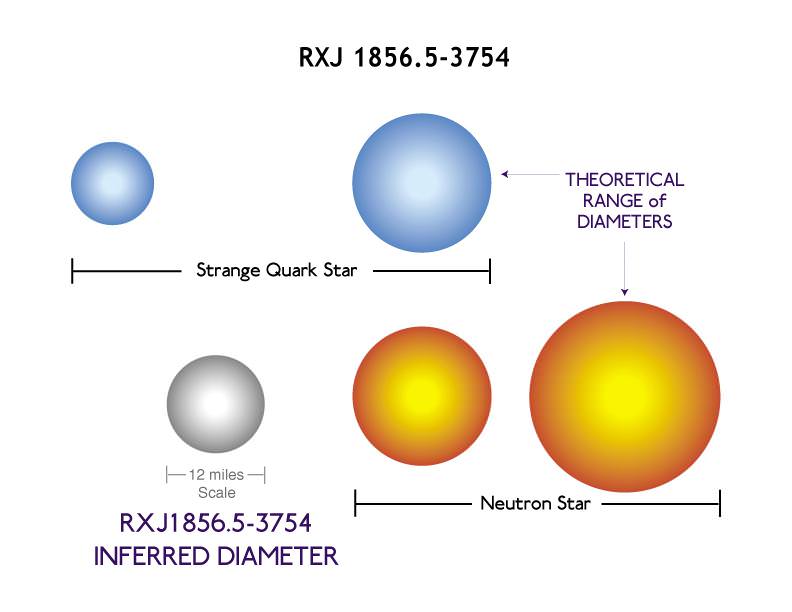
Another distinct feature should be a size that is smaller than most neutron stars. One strange star candidate is RXJ1856, which appears to be a neutron star, but is only 11 km in diameter. Some astrophysicists may have muttered hmmm… that’s strange on hearing about it – but it remains to be confirmed that it really is.
Further reading: Negreiros et al (2010) Properties of Bare Strange Stars Associated with Surface Electrical Fields.


“However, since the LHC hasn’t done any such thing, it’s reasonable to think that strange stars probably don’t form this way either.”
Hey. it ain’t at full power yet! But I’m hoping that the theory that is would many more times the power and luminosity than the LHC to make stable stranglets is right. Our confidence’s is high.
electrons flow around the surface of neutron stars and topological insulators. Perhaps an electron star briefly forms by a condensed matter state before total anti-matter annihilation of particle pair production occurs, leaving or forming a black hole? Quantum and macro states seem proportionally related to charges and their distances apart explainable without gravity by condensed matter plasma physics. Black holes have no size ranges, so perhaps all stars including tiny dwarf stars are in the same charged plasma medium pervading the universe, and are forming the early big-bnags missing neutron galaxies, as all normal galaxies having black hole central cores build up denser neutron star concentrations over time.
The conspiracy theory was that you turn the LHC on and the world ends. Didn’t happen – no need to give the idea more oxygen 🙂
Wow. That’s a lot of strangeness!
Do we know enough to reasonably confer that these Strange Stars would be the final, and highest density objects before encountering Black holes?
Also, I’m unsure of how such an object would become a Black Hole if it were to eat enough mass. I have difficulty envisioning how all this works and looks to an outside observer. Would the event horizon grow from within and the star’s surface disappear behind it? Just curious..
Question: Where do the electrons come from?
I mean, firstly they all merged with protons to build neutrons. And now it has just happened that the neutrons cracked up into “strange matter”. I don’t see from where the electrons should come back.
Or does it have to do with the appearance of the strange quarks (which weren’t around before, either)? But no, this cannot be, since both down and strange quarks have the same charge (-1/3 e), and the strange quark should be made from a down quark (in neutrons we had up:down:strange = 1:2:0 …. in strange matter this is according to the text 1:1:1, so down quarks seems to have changed into strange quarks….).
I see, a “long” time has gone by since I had any particle physics lectures. 😉
Still: Where do the electrons come from?
@Dr Flimmer
Welcome comments from the floor, but my understanding is that the transition from neutron to strange matter may release an equal number of electrons and positrons which potentially annihilate leaving a nett zero sum behind.
If the whole hypothetical quark star model is correct, I understand that the electrons that remain were already there just hanging around, having been excess to (or never engaged in) the proton+electron = neutron process.
Hope that’s helpful (and correct).
If it happens, it will inaugurate a pleasing (and hopefully informative) state between microstate baryonic matter and the more or fully macrostate black hole.
Aren’t we all?
AFAIU the manner of transition would depend on how much of a coherent state the system is in before and after. Does it transit between states or does the system trajectory go between many states?
And any coherence could have implications for how the whole mess couples to semiclassical theory (“QM+GR”). But in general I believe since the event horizon is one of an ever dimmer surface (ever fewer photons seen by outside observers when looking at infalling objects), such a black hole transiting object would look just like that: the star would eventually dim out.
The question then becomes, in my mind, how fast that goes. Unless there is some really funny stuff going on to make event horizon growth comparably slow, I would presume the infalling matter would simply control the rate of dimming.
It hits me that I’m sloppy again: “event horizon growth” usually refers to its area growth. But that wasn’t what I was describing, or at least not all of it.
What should we call the gradual (or not) concentration of space-time curvature that happens when it instantiates? “Event horizon conception”?
As for the electrons, yes, that is curious.
The reference mentions that there is a “diminishing quark chemical potential toward the stellar surface, which renders the (negatively charged) strange quarks less abundant.” (So out goes my idea of strong coherence.)
To maintain charge neutrality then electrons set up an outer sheet as part of a dipole layer. The further references are old or pay-walled, but I take it the quark matter state still applies, merely “less strange”. (&_&)
So the electrons could come from wherever, within a Debye length.
Actually there is a further complication there, as “the increase in gravitational mass of strange stars, resulting from the energy associated with the electric dipole layer on the surface, is very small. We can conclude then that only when the star possess a net charge one might find a significant contribution to the gravitational mass, due to a macroscopic extension of the electric field outside of the star.”
So if the star _isn’t_ neutral, it also acquires gravitational potential besides the electrical, if I understand correctly. It will then have a further energy loss when it slurps up those electrons. Should we think of that as a “Debye-Einstein” effect?
“So the electrons could come from wherever, within a Debye length.”
Except that on further cogitation, if it is still quark matter, Steve’s description must then be correct; the bulk of these electrons must be part of the hydrogen to neutron to quark matter system in the first place.
@ Steve Nerlich
So we agree. IIRC, I read somewhere that even “normal” neutron stars are surrounded by some kind of an atmosphere which is basically made up of iron nuclei and most likely enough electrons to keep charge neutrality.
The question that follows then, of course, is how likely it is that the positive ions and the electrons build up a double layer with different rotational velocities.
Maybe the preexisting magnetic field of the precursor star could play a role. Due to the compression of the star’s core into the neutron star the magnetic field strength has already grown by many orders of magnitude.
I am not quite sure if this could aid the formation of a differentially rotating double layer. But even if it does, I somehow doubt that this would increase the magnitude of the magnetic field, since this would lead to some kind of a runaway effect (kind of perpetual motion 😉 — or it could finally disrupt itself in some of these very massive explosions one can observe on neutron stars). I guess that the double layer would somehow weaken the preexisting field (thinking of Lenz’s law). This would lead to some kind of stable configuration between the magnetic field strength and the possibly induced double layer.
However, since the preexisting field should be a dipole field to leading order, I am not sure if this could really build a double layer near the surface.
Maybe it’s just some centrifugal effect; or some other weird things due to the (very) strong gravitational potential. 😉
Strangelets caused a bit of a stir in the 1990s when the RHIC at Brookhaven came on line. This was echoed by later concerns of black holes being produced in the LHC, due to theories which connect extra dimensional physics at these energy scales with small quantum amplitudes for black hole or AdS physics. To start these concerns were never worth the worry. Cosmic rays at far higher energy slam into the Earth’s atmosphere, the moon, Mars, the sun and so forth. If these processes were the dominant or lower energy process then they would have already happened. If strangelets were the preferred state of quark matter, being the lower energy configuration, the universe at large would have long ago assumed that configuration, in fact right after the inflationary period.
I will try to give a description of this without getting into too much math territory.
The issue with strangelets is mildly troubling from a theoretical perspective. The problem is that the strangelet configuration is at a lower energy than standard up-down quark structures for a large ni-quark system bound by gluons. The issue is whether this could catalyze the existence of more strange matter. The problem is set up if you think of one state of matter having two slots for up and down quarks (u, d) and another state of matter containing (u, d, s) quarks. Both of these have a ladder of states which permits two quarks of the (u, d) and (u, d, s) varieties in spin up and down ordering. This is the Pauli exclusion principle, which operates much the same as with how atomic shells are filled with electrons. The up quark has charge 2e/3 and down quark has charge -e/3, for e the quantum of charge of an electron. The strange quark has charge -e/3. The strange quark has a mass ~ 130MeV, which is considerably more massive than u and d quarks ~ 10MeV. So if you have only three quarks, the energy configuration for particles in the two slots is lower than for the three slots, because the strange slot is raised up by that 120MeV or so. However, if you have some configuration of matter with lots of quarks then as you start filling up the two quarks “slot system,” the top of the two-quark bin system fills up faster than the three quark bin system. The top of water fills up a narrow glass faster than a glass with a broad base, even if you put some object that displaces liquid meant to simulate the energy step of the s-quark mass. Then at some point, around 50 quarks or so the (u,d,s) configuration has a lower energy than the (u,d) configuration. So quark gluon plasmas should for a large number of quarks preferentially convert the up quark into strange quarks, which is the converse of the decay mode s – -> u + electron or other lepton.
RHIC experiments with gold nuclei collisions have identified this state of quark-gluon plasmas. So the centers of neutron stars, or the state of quark stars should be strange matter (strangelets). The question emerges as to why this does not happen with ordinary nuclei. A large nucleus with lots of protons and neutrons is very dense and similar to the state of matter in a neutron star. So there should be some probability that a nucleus would spontaneously convert itself into a strangelet state. Some arguments were presented that a strangelet would act as a sort of catalyst, so an ordinary nucleus that interacts with a strangelet would also become a strangelet. Yet the world we observe indicates this is pretty seriously forbidden. So there is a bit of a question lurking in the wings. I think the answer to this question might be with the duality or correspondence between QCD and the anti-deSitter spacetime in 3 dimensions. This AdS spacetime can be converted into something called a BTZ (Banados, Teitelboim, Zanelli) black hole. There is a mass-gap, whereby the BTZ black hole does not exist. This probably means that for energy or pressures below a certain value the gauge charge is too large for the corresponding object to exist.
LC
@DrFlimmer
Well, all that stuff about dipole layers is exactly the subject of the ‘further reading’ article – I defer to their expertise (and math).
A neutron star would be expected to already have an intrinsic spin before it goes strange. It’s common in celestial bodies that an atmosphere drags behind a planet/star etc, so a differential rotation of a strange star and its ‘atmosphere’ seems entirely plausible (albeit the entity itself is entirely hypothetical).
@Uncle Fred
Sorry, I missed your question. Well, we don’t know enough to be sure strange stars even exist, so whether they are the last stage before the information loss associated with black hole formation is unknown.
Black hole formation is all about reaching a critical density. Keep piling mass on and the core experiences the most compression, so the core should hit that critical density first.
How this might look to an outside observer is a thought experiment in general relativity. From your external frame of reference, clocks will appear to run extremely slowly near its surface. Also, the light it emits will become extremely red-shifted, fading slowly towards black. So, you shouldn’t expect to ‘see’ a sudden and dramatic change – any information about those last moments will come to you only slowly and faintly. Not a bang, but a whimper.
Unless of course, there’s a huge X-ray emitting accretion disk spinning around it – in which case, you’ll die 🙂
Mr. Crowell! You are a treasure, sir and I hope you will bear me with this one. I have understood the Cosmic Rays are sooo much more powerful anyway to be a Very Good Argument against either the RHIC or LHC ending the world. But it is all about the Tev? What about luminosity? How does does the luminosity of our machines compare to the naturally occuring showers of Tev beasties?
That doesn’t explain the paper strange (negative charge) surface depletion though, you would need more electrons. But the quark matter conversion supply or deplete the majority of the electrons AFAIU. (LBC made it explicit.)
[And I believe I misread Steve on this point. Oh, well.]
@ LBC: Very pedagogic walk through, thanks!
D’oh, I knew in returning I forgot to point out that the article lacked something. Thanks again, LBC!
@ Vanamonde:
As for the energy, at some point an accelerator could theoretically overachieve the cosmic ray (CR) energy, since it is dampened by various factors over the vast distances it travels. Most prominently they interact with the CMB photon gas, as I understand it. (The GZK limit.)
OTOH, then you have still the CR source region to compete with. In practice, there is some way to go to the first goal already (~ 10^1 vs 10^20 TeV).
As for the luminosity, there are papers comparing luminosity as it makes for a good risk calculation. The thing is that billions of years of CR make up for the LHC luminosity, the above reference finds 10 orders of magnitude more CR interacting with our sun alone than for the LHC at present luminosity. (9 orders after upgrade.)
And that is for one normal star alone. We have plenty of those in the observable universe.
Might the ‘excess’ of electrons at the surface be down to the fact that they a point-like objects with no interior structure whereas the neutrons are a 3-quark particle?As the neutrons become even more compressed they ‘release’ their electrons [becoming protons for a short time??] and the resulting protons and neutrons then ‘break down’ into their constituent quarks. Just a thought.
The occurrence of electrons is from channel or amplitude s – -> u + e. The strange quark has a charge –e/3 and the up quark is 2e/3. Under the extreme pressures in the centers of these compact objects the inverse process occurs. A neutron is a baryon quark triple (u,d,d), for the down quark charge –e/3. So in the quark-gluon plasma we may think of triplets of quarks are being “melted” neutrons (u,d,d) or metled Lambda^0 particle (u,d,s). The conversion of a triplet (u,d,d) to (u,d,s) results in no electron excess, but if there is a boundary layer in the star where three quarks at higher pressure are (u,d,s) then a slight reduction of pressure will result in (u,u,d) + e or a melted proton plus and electron. This “proton melt” is unstable and the electron can be absorbed into the up quark to form a down quark plus a neutrino. A quark star or a neutron star with a quark-gluon plasma at the core will as a result have an energy gradient for an electron. This is particularly the case if it has a crust composed of proton-electron matter, or degenerate iron.
Particle physics above the electro-weak unification scale has a conformal renormalization group (RG) flow. RG flow is some physics which occurs for zero mass particle where the gauge potentials scale continuously with transverse momentum. The word conformal refers to both the Cauchy-Riemann conditions in complex variables, which in higher complex valued dimensions is called a holomorphic condition, and is also connects with conformal symmetry in general relativity. I mention this for those who might be interested in looking it up. This conformal RG flow breaks down at lower energy due to the onset of mass, which is the low energy vacuum condition induced by the Higgs mechanism. Above the unification scale, masses at around 150 GeV, or transverse momenta at ~ 1TeV the Goldstone boson or Higgs is recovered and gauge interaction physics is in a way “liberated” from the Higgs. The LHC is set to find the Higgs field this way and to examine particle physics in the RG flow domain. Because of this it is theoretically possible, which can be tested, that particle physics at the 10TeV range carries feature of physics all the way up to the string and quantum gravity limit. The scaling is logarithmic, so even with a reduction of 14 to 15 orders of magnitude in energy, the attenuation in these amplitudes is only a few orders of magnitude or so. This also connects up with the UV/IR correspondence in the holographic principle.
Cosmic rays interact with matter on any celestial body, including the Earth’s atmosphere. Such interaction do produce Lambda hyperon particles. If there was a strangelet autocatalysis then everything would be strange matter now. So there is a fundamental obstruction to this sort of process. It is not possible for a quark-gluon system smaller than about 150-200 quarks (I said 50 above and I was thinking of nucleons) and energy below 100 GeV or so. So it requires the high pressures and temperatures found in high mass neutron stars or quark stars to generate strange matter.
LC
Um, yes, that would be the result it seems to me, and LBC seem to confirm this.
In any case there must be a free energy change for the quark/electron system, if one can interpret it as a “quark chemical potential” (see the quote from the paper). Pressure change (inherited from gravitational potential energy change) would indeed be the obvious culprit for the free energy change, but what do I know about bound quark systems? :-~
[Paywall references, let me enumerate all the ways I hate you … eh, better not, or we will be here indefinitely.]
The physics is in a three layer sandwich. With the left being close to the core a triplet of quarks are
—(u,d,s)—|—(u,d,d)—|—(u,u,d)—
0 – – increasing radius – ->
where on the far left is the “melt” or quark gluon plasma “triplet.” This triplet would construct a Lambda^0 hyperon by itself, but where the quark triple is shown here for ease of discussion. The middle layer is the neutron material layer. This layer I am somewhat aware of is itself rather complicated, but FAPP it is a gas or fluid of neutrons. Then there is the layer of degenerate iron as a crust, where the (u,u,d) indicates the presence of protons. So there are three boundary layers here. The top one will involve transitions between up and down quarks, with d – -> u + e + anti-neutrino or u + e ? d + neutrino. So as material feeds onto the quark star, such as an accretion disk, up quarks along with electrons are converted into down quarks. The third boundary layer involves the conversion between d and s quarks. However, the d quark in the middle layer first converts to an up quark d – -> u + e + anti-neutrino, which is an unstable or excited state (the ball pushed a bit up a hill), and the e is absorbed again to produce the strange quark (the ball rolls down the larger back side of the hill). This involves something called semi-leptonic processes and is involved with why the s is called the strange quark. The net effect is a transport of electrons from these charge separation as computed by pressure gradients in the paper. The conversion of neutron matter into the strange matter generates current flows and available electrons for this charge separation.
LC