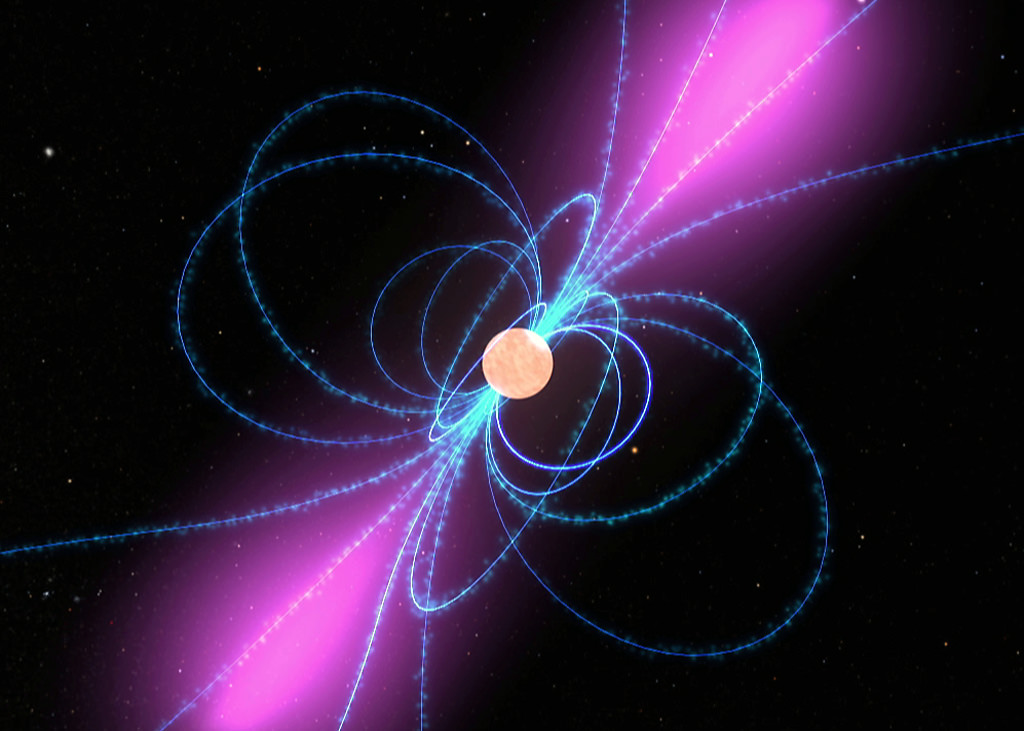
This illustration shows a pulsar’s magnetic field (blue) creates narrow beams of radiation (magenta). Image credit: NASA
How do you detect a ripple in space-time itself? Well, you need hundreds of precision clocks distributed throughout the galaxy, and the Fermi gamma ray telescope has given astronomers a new way to find them.
The “clocks” in question are actually millisecond pulsars – city-sized, sun-massed stars of ultradense matter that spin hundreds of times per second. Due to their powerful magnetic fields, pulsars emit most of their radiation in tightly focused beams, much like a lighthouse. Each spin of the pulsar corresponds to a “pulse” of radiation detectable from Earth. The rate at which millisecond pulsars pulse is extremely stable, so they serve as some of the most reliable clocks in the universe.
Astronomers watch for the slightest variations in the timing of millisecond pulsars which might suggest that space-time near the pulsar is being distorted by the passage of a gravitational wave. The problem is, to make a reliable measurement requires hundreds of pulsars, and until recently they have been extremely difficult to find.
“We’ve probably found far less than one percent of the millisecond pulsars in the Milky Way Galaxy,” said Scott Ransom of the National Radio Astronomy Observatory (NRAO).
Data from the Fermi gamma-ray space telescope, which started collecting data in 2008, have changed the way millisecond pulsars are detected. The Fermi telescope has identified hundreds of gamma-ray sources in the Milky Way. Gamma rays are high-energy photons, and they are produced near exotic objects, including millisecond pulsars.
“The data from Fermi were like a buried-treasure map,” Ransom said. “Using our radio telescopes to study the objects located by Fermi, we found 17 millisecond pulsars in three months. Large-scale searches had taken 10-15 years to find that many.”
Ransom and collaborator Mallory Roberts of Eureka Scientific used the National Science Foundation’s Robert C. Byrd Green Bank Telescope (GBT) to find eight of the 17 new pulsars.
Right now astronomers have only barely enough millisecond pulsars to make a convincing gravitational wave detection, but with Fermi to help identify more pulsars, the odds of detecting these ripples in space-time are steadily increasing.
Ransom and Roberts announced their discoveries today at the American Astronomical Society’s meeting in Washington, DC.

Wouldn’t “city-sized, sun-massed stars” be a better description of pulsars/neutron stars?
So, we are clocking pulsars, now? Gravity waves, your time’s up!
D’oh. Good catch DrFlimmer! I’ve fixed the mistake.
Oh, so that’s why pulsars haven’t been as hot as man-made experiments to detect gravity waves!
@ DrFlimmer:
Seems it’s time for gravity to shore up its theory.
This will be very difficult to detect I should think. The “time-time” component of the curvature of a gravity wave is larger, by a c^2 factor, than the spatial parts. The metric is a flat spacetime metric g_0 perturbed by a term h, and the traceless part of h will interact with material by the equation
&^a&_ah_{cd} = (16piG/c^4)T_{cd},
for the small letters abcd are indices running over the spacetime coordinates. For c = d = 0 this is a time-time part, and there is an over c^2 multiplied here. However, the thing to look at is the resulting (16piG/c^2) is very small. You have
G = 6.7e^{-11} m^3/kg-sec^2
c = 3e^8m/sec
so the coupling is 3.7e^{-26}m/kg, which is pretty small. The left hand side involves curvatures smaller than the square of the diameter of an atom.
So this is a pretty serious challenge.
LC
There have been a few international projects for a while observing pulsars to detect gravitational waves, http://bit.ly/5z7UOG is among them. Hopefully the extra pulsars found with the Fermi telescope will help.
LBC… you are such a scarecrow.
At any point, the equation you ran through doesn’t make any argument as to how easy or hard the challenge of detecting gravity waves will be. Nor does it prove the illusivness of them.
It’s obvious you don’t know much about using the linear equation you gave as an example (which by the way, only truly works when one considers ST is flat… which it isn’t).
In other words, it can only be an approximation. Most of all, it has no real significance outside simple analysis.
For instance your example can basically be used to show a wave moving away from a point, or a wave moving towards a point. Since this would be a ‘flat’ vector.
For ST, everyone is going to use Einstein’s equations of general relativity. Using the metric tensor for the curvature of ST.
It is obvious I don’t know much about using the linearized equation either. But surely you would expect to use it as we measure gravity waves in the weak gravity of Earth?
Anyway, that seems to be exactly the way Frederick Jenet of the Pulsar Timing Array project does it.
What they end up with isn’t a constraint on local curvature change, which presumably is that LIGO would have to contend with, but a relation on timing residuals for the pulsar signal as affected by gravity waves en route to Earth.
“To detect a general background of gravitational waves, astronomers would need to monitor 20 millisecond pulsars for five to 10 years, with the arrival time of the radio waves determined to an accuracy of 100 nanoseconds, Jenet estimates. Recording gravitational waves from a pair of merging supermassive black holes would require five pulsars with radio wave arrival times known to an even higher accuracy, 10 nanoseconds, he adds.
“We currently have about 20 millisecond pulsars, but only five of these can be timed to the needed precision,” he notes. Astronomers need to find more millisecond pulsars in order to conduct the proposed search.
The pulsar technique, Jenet says, is best suited for recording long-period gravitational waves — those with a period of months to years — because those waves would induce the largest fluctuations in the arrival time of the radio waves. Intriguingly, long-period gravitational waves are preferentially generated by pairs of the most massive black holes — the giant beasts that lie at the center of galaxies.” [Science News.
So the photons can be stretched over months by each GW. Looking at his presentation, large BH binaries/mergers should be detectable out to 100 Mpc, perhaps 1 Gpc.
How difficult is it to measure arrival times to 10 – 100 ns? I dunno, but 1977 a time to digital converter used at CERN had a resolution of 1 ns. Todays computers are clocked at 1 GHz, or 1 ns period, with a phase jitter of 0.004 ps for low-noise clocks. So the capability of off-the-shelf electronics is surely in that range.
Torbjorn,
Thanks for providing some links to what I was referring to. Very good find!
The links you provided are using the mathematics I stated would be used (Einstein’s General Relativity; consisting of many equations).
In fact, the 5th slide you provide which covers gravitational waves, uses the equation with both the metric tensor and Einstein tensor to display ripples in the fabric of ST.
In any case… they aren’t using the equation LBC cut and pasted in order to find the waves, or to prove how difficult they would be to find… which by itself doesn’t pass the giggle test.
@ Aodhhan: The left hand side of this equation is the Ricci curvature for weak gravity. The Ricci curvature in this case reduces to
R_{ab} = &_c&^ch_{ab} = (16piG/c^4)T_{cd},
for &_a the partial derivative. Gravity waves we detect are likely to be very weak. Weak gravity reduces to linear field equations, where for very weak gravity you recover Newtonian gravity. The term h_{ab} is a metric perturbation term added to a flat spacetime metric. There are two transverse degrees of freedom for the wave equation, or solutions to h_{ab}, which are the two orthogonal directions of polarization for the weak field. Of course we could detect very strong gravity waves from a nearby collision between black holes, but if so we could also end up dead and the Earth reduced to a pile of rubble.
The main point is the left hand side is the gravity field and the left hand side involves the field density of mas-energy and momentum. The coupling constant (16piG/c^4) is very small. This is why the LIGO is set up to detect the oscillating motion of weak gravity waves which move the arms of the interferometer to less than an atomic dimension over the entire length of the Fabrey-Perot-Michelson interferometer.
This linearized field equation is a standard expression you will find in any text on gravitation and general relativity. This is not something of my invention, but something which is well established and known. Weinberg’s book “General Relativity and Cosmology” has a section on this. I would advise looking this up and learning it for yourself.
If someone is wrong on something you can point that out in a civil manner. If you are not sure it is a good idea to check it out first before you make insulting statements and claim something is wrong when in fact it is right and well known. I am not sure why, but it is clear there is some sort of enmity here on your part, which is odd, because I have not the foggiest idea who you really are.
Lawrence B. Crowell
erratum: I made a mistake in writing:
The main point is the left hand side is the gravity field and the left hand side involves the field density of mas-energy and momentum.
when it should read
The main point is the left hand side is the gravity field and the right hand side involves the field density of mas-energy and momentum.
LC
My point is, the information you are pasting has no real value to this subject, and it is obvious you have no real knowledge of what you are pasting.
…and now, you bring up something totally different from what you pasted earlier.
Trust me… this is a subject you don’t have to educate me on. I didn’t say your formula didn’t exist… I gave an example of what it is good for, and none of them works into this subject. When you see me post something, it is my own work. I don’t cut and paste things I’ve searched for on the web.
All you are is a scarecrow… you search for the subject, then cut and paste something you believe is correct, in some lame attempt to make people believe you know everything. However, I must admit… when you do add your own thoughts… at times they are so whacked it makes me laugh.
If you want to do something which is actually on subject. Work out the formulas for monitoring the timing change of an array of pulsars to detect gravitational waves. Or perhaps, show the minimum number of pulsars it will take to create an array.
I think working out formulas for this and the like is a bit beyond a brief blog post! Experimental test for gravity waves is related to but not directly my forte. My comment was to illustrate how small an effect this is, and how difficult I think this might be. That was all I was saying, and I gave a bit of physics for that.
As for an array of pulsars this calls into question what is meant by that. To me an array implies a set of detectors which measure a distributed wave. Many pulsars spread across a galaxy would make for a long duration detection of a gravity wave! On the other hand many single detection of independent events by an array is just a repeated set of observations or experiments. I have not calculated anything, but I might conjecture it would take at least five pulsars to minimally characterize a gravity wave, which in a spacetime sense acts as a 4-dim tetraheron that “triangulates” the spacetime. In effect this is a spacetime version of a land survey. This is a type of geodesy or survey that maps time as well as space. A time shift in one pulsar might suffice in indicating the presence of a gravity wave, but you will have no information concerning its direction of propagation or polarizations.
You appear to be in keeping with a number of physicists I know who treat their students like crap, and compete with or have constant fights with others. You strike me as a rather adversarial individual. One of the most important educators in the last century was Mr. Rogers, you know “Mr. Roger’s Neighborhood.” If you have young children it gives you a good excuse to watch reruns of this program. There you will learn lessons, far more valuable than any astronomy and physics course, on how to treat others with respect and kindness, even if you disagree with them on something, and avoid throwing insults at people. In taking this to heart you might find that life is somewhat happier.
Cheers LC
Torbjorn Larsson OM wrote: “large BH binaries/mergers should be detectable out to 100 Mpc, perhaps 1 Gpc” using the pulsar technique.
Setting the detection limit at 1 Gpc would allow us to monitor the Fornax, Coma, Virgo and Perseus galaxy clusters( among others) for signals. The large number of SMBHs in these clusters may bode well for the detection of a SMBH binary radiating gravity waves in that vast volume of space.
LBC…
You’re a terrible tap dancer. But a great scarecrow on the yellow brick road.
You have no idea what you are posting, and what you posted makes no sense. Give it a rest. Everything you post only makes you look more ignorant to people who really understand these things. Yet… at times, it is a great laugh.
I’m not adversarial. However, I’m not going to let someone talk BS around here, when people come here for information. Although, since I don’t see many people respond to your rantings (except maybe a few new individuals)… most have already figured out you’re nothing but a patzer.
Frankly, what you posted on January 5th, 2010 at 6:29 pm makes me suspect you don’t know as much as you claim. That you did not recognize G_{ab} as the d’Alembertian on the metric perturbation h_{ab}, and then further said I was not using a metric makes me suspect you are not as enlightened about relativity as you claim.
I don’t think I am talking BS. I just mentioned the coupling constant of gravity as being weak. Then you come on as some beluga with insults and rants. WTF?! Nobody appointed you to be a police officer here. Anyway if you are going to call me a scarecrow, then I will start calling you trash.
LC
@Aodhhan: What are your scientific credentials? You seem very adept at criticism of others comments, but rarely offer any of your own theories or opinions. I come to this site to learn more about astronomy and science, not to read diatribes attacking others. I eagerly await reading something from you that I can learn from, since I don’t have a background in astronomy/astrophysics/physics/mathematics.
Sad to see this sort of mud-flinging taking place between adults here on UT. (and, without naming names, it’s clear to any objective reader exactly who it was introduced this level of invective!) I endorse what William928 says. We come here to learn. Being critical and yet courteous should not be beyond the ability of any contributor.
@ Aodhan:
Eh? AFAIU it is reducible to one tensor equation, Einstein’s field equations: curvature of spacetime = stress-energy, …
… which is precisely the equation LBC quoted.
Besides, they use verbatim LBC’s linearization, the exact form of the full theory doesn’t matter as long as you get that correct. (Not only the method, but the result, of course.)
@ Jon Hanford:
Thanks, that is excellent news! I dunno when and if the 10 ns technique will be used though.
To be fair the Einstein field equation
R_{ab} – 1/2Rg_{ab} = 8piGT_{ab},
here with c = 1 has 16 components. The symmetric tensors R_{ab} are 4×4, and this symmetry R_{ab} = R_{ba} means the off-diagonal components are equal, which is 6 in total. So there are on a component level indeed 10 field equations.
Thanks for posting that reference.
LC
@ Torbjorn Larsson
Just a question. On some of these blogs links can be embedded with text using a command similar to
[link: URL link] text[/link].
Is that how one can embed things here? I don’t see a help guide for doing that here.
LC
LBC, I have used HTML [a:href:”URL link”] text [/a].