Brian Koberlein, Author at Universe Today

November 17, 2014December 23, 2015 by Brian Koberlein

Where’s All The Antimatter?

Protons, neutrons, electrons - particles in an atom.

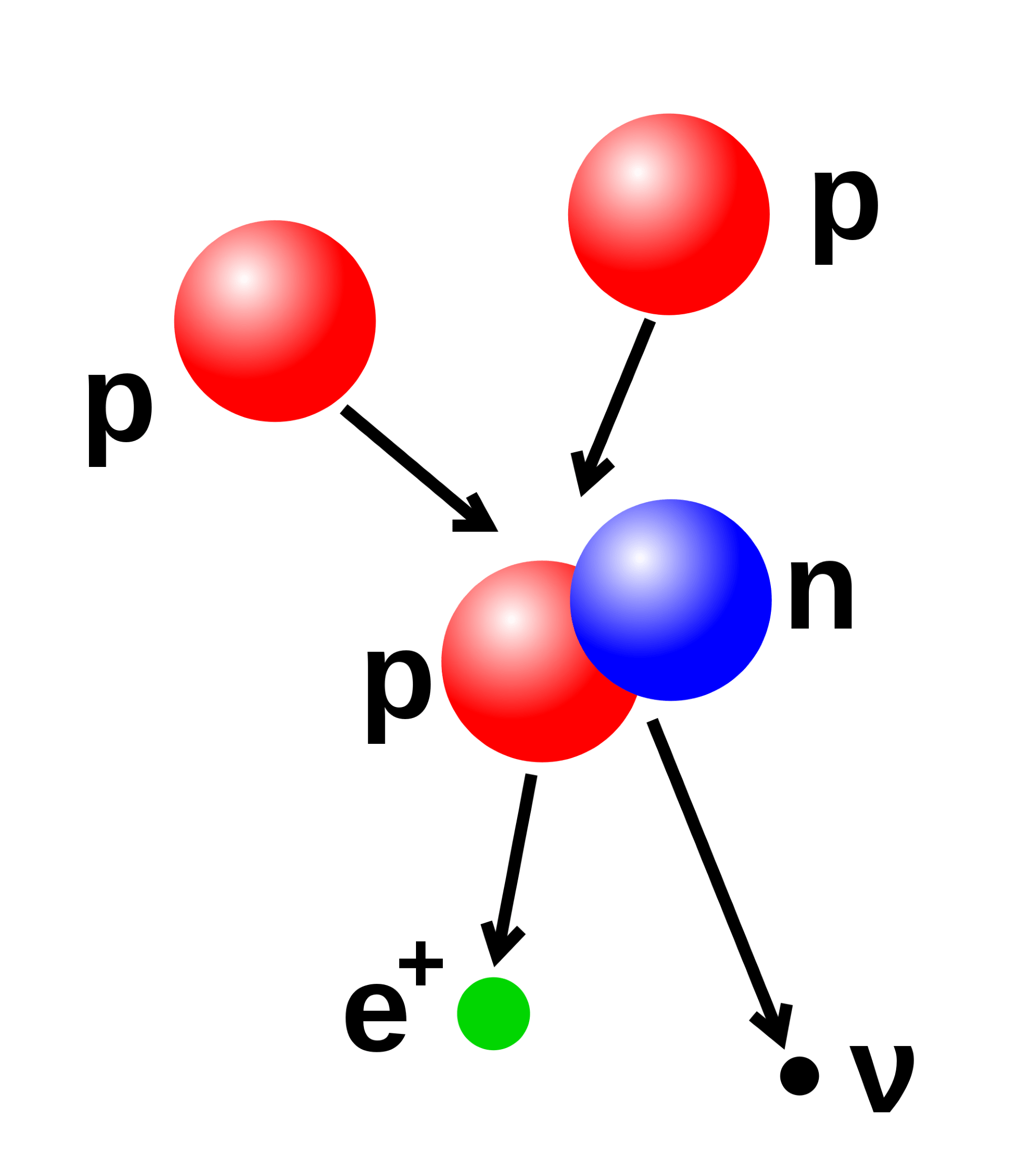
One of the biggest mysteries in the Universe is the fact there there’s matter, and not antimatter. Where did it all go?

When we look around, everything we can see is made of matter. For every type of matter from electrons, protons and quarks there is a similar type of matter known as antimatter. So why aren’t there piles of antimatter rocks, cars and chocolate bars just lying around? Why does Scotty always have a little extra kicking around in his liquor cabinet? And where do I get mine?

The primary difference between matter and antimatter is that they have opposite electric charge. Which seems pretty mundane. The negatively charged electron has an antiparticle known as the positron, which has a positive electric charge.

Anti-protons have a negative charge, and are just flat out grumpy. We’ve been able to create these particles in the lab, and have even been able to create small amounts of anti-hydrogen consisting of a positron bound to an antiproton, when examined closely there’s were shown to have a goatee and a little colorful sash or dagger.

When we create particles in accelerators such as the Large Hadron Collider, we seem to get equal amounts of matter and antimatter. This suggests that when particles were formed soon after the big bang, there should have been equal amounts of matter and antimatter.

Particle Collider — Large Hadron Collider (CERN/LHC/GridPP)

But the universe we observe is only made of matter, and… here’s the best part… we have no idea why. Why didn’t the matter and antimatter completely annihilate each other? How come we ended up with a little more matter? This delightful mystery is known as baryon asymmetry.

We do have a few ideas, and by we, I mean some giant brained crackerjacks have come up with a few plausible options. The most popular is that somehow antimatter behaves a little differently than matter. This could cause an imbalance between matter and antimatter. After particles collided in the early universe, there would still be matter left over, hence the matter we observe.

Another idea is that the observable universe just happens to be in a region dominated by matter. Other parts of the multiverse could have observable universes dominated by antimatter. Baryon asymmetry is one of the big mysteries of modern cosmology.

Zero Gravity Flight — Stephen Hawking, weightless (courtesy Zero Gravity Corporation)

There is an even crazier idea. Antimatter might have anti-gravity. In other words, an atom of anti-hydrogen would fall up instead of down. If that is the case, then matter and antimatter would repel each other, and you might have matter universes and antimatter universes that are forever separate.There have been some initial experiments to test this idea, but so far there have been no conclusive results.

What do you think? Where’s all our antimatter and how do we track it down? I’d sure love to bring some home and show my friends…

And if you like what you see, come check out our Patreon page and find out how you can get these videos early while helping us bring you more great content!

November 10, 2014December 23, 2015 by Brian Koberlein

Why Doesn’t The Sun Steal The Moon?

On Sept. 18, 1977, Voyager 1 took three images of the Earth and Moon that were combined into this one image. The moon is artificially brightened to make it show up better. Credit: NASA

The Sun has so much more mass than the Earth. So, so, so much more mass. Almost everything in the Solar System is orbiting the Sun, and yet, the Moon refuses to leave our side. What gives?

The Sun contains 99.8% of the entire mass of the Solar System. It looks to us like everything seems to orbit the Sun, so why doesn’t the Sun capture the Moon from Earth like a schoolyard bully snatching the Earth’s lunch money. That would make sense right? It all fits in with our skewed view of social hierarchy based on an entities volume.

Good news! It’s already happened, In a way. The Sun has already captured the Moon. If you look at the orbit of the Moon, it orbits the Sun similar to the way Earth does. Normally the motion of the Moon around the Sun is drawn as a kind of Spirograph pattern, but its actual motion is basically the same orbit as Earth with a small wobble to it.

The Moon also orbits the Earth. You might think this is because the Earth is much closer to the Moon than the Sun. After all, the strength of gravity depends not only on the mass of an object, but also on its distance from you. But this isn’t the case. The Sun is about 400 times more distant from the Moon than the Earth, but the Sun is about 330,000 times more massive.

If you’re up for some napkin calculations, you little mathlete, by using Newton’s law of gravity, you find that even with its greater distance, the Sun pulls on the Moon about twice as hard as the Earth does.
So why can’t the Moon escape the Earth?

In order to escape the gravitational pull of a body, you need to be moving fast enough *relative to that body* to escape its pull. This is known as the escape velocity of the object.

It takes two to tango. The moon’s gravity raises a pair of watery bulges in the Earth’s oceans creating the tides, while Earth's gravity stretches and compresses the moon to warm its interior. Illustration: Bob King — It takes two to tango. The moon’s gravity raises a pair of watery bulges in the Earth’s oceans creating the tides, while Earth’s gravity stretches and compresses the moon to warm its interior. Illustration: Bob King

So, yes, the Sun is totally trying to rip the Moon away from the Earth, but the Earth is super clingy.
The speed of the Moon around the Earth is about 1 km/s. At the Moon’s distance from the Earth, the escape velocity is about 1.2 km/s. The Moon simply isn’t moving fast enough to escape the Earth.

Man, those numbers sure are close. I wonder if we could kickstart a rocket to stick on the side? So, even though the Moon can’t escape the Earth, it is gradually moving away. This is due to the tidal interactions between the Earth and Moon, which we talk about another video we’ll link at the end of this one.

So even though the Moon will never escape the Earth, it will continue to move away. So, what do you think? What kind of devious project should we start to get the Moon that little boost so it finally escapes the clingy Earth and all its clingy Klingon clingyness? Tell us in the comments below.

And if you like what you see, come check out our Patreon page and find out how you can get these videos early while helping us bring you more great content!

October 20, 2014December 23, 2015 by Brian Koberlein

What Would A Black Hole Look Like?

Artistic view of a radiating black hole. Credit: NASA

If you could see a black hole with your own eyeballs, what would you see?

Here on the Guide to Space production team: We love everything about Black Holes.

We like how they’re terrifying and completely conflict with our day to day experience of how stuff should work. We like how they completely mess you up before absolutely tearing you pieces, and we like how they ruin time and space and everything nearby.

We like them so much, we even enjoy giving them cute nick names like “Kevin”.

So I’m now going to show you images and animations of black holes.
Should you find this either too exciting or terrifying and need a breather I suggest you pause the video and walk around the block and try not to think about how absolutely terrifying these things are.

Those are just the artist’s illustrations, who’ve no doubt been awe inspired in the same way the rest of us have… but those people have never ACTUALLY seen one. Have they?

Is that what a black hole would really look like? Or are these just pictures of lasercorns?
I’ve got good news!

Here’s a picture of a real black hole. Can’t see much? That’s because it’s more than 25,000 light years away. It’s got 4 million times the mass of the Sun, and it’s still a tiny dot.

So, how do we know it’s there? The answer is awful. Even if we can’t see them directly, they make such a giant mess of things in their neighborhood we can still figure out where they are.

For an actively feeding black hole, we see a disk of material surrounding it.

Quasars are the jets emanating from active black holes, and we see them billions of light-years away. As you get closer, this area would get brighter until it was like you were close to millions of stars. The radiation would be overwhelming. Closer and closer, there would be region of total darkness, that’s the black hole itself.

For non-active or “sleepy time” black holes, we’d only see the distortion of light around them as light is bent by gravity. As you got closer and closer, there’d be less light coming from the area around the black hole. No photons can be reflected by it. You’d then pass a region called the photon sphere, where light is orbiting the black hole. You’d see the whole Universe as a swirling jumble of mixed up photons.

Next the event horizon, where light can’t escape. You could look out into the Universe and see the distorted light coming from everywhere, but the singularity itself would still be dark. Is it a single point, or a sphere? Astronomers don’t know yet.

A new telescope is in the works called the Event Horizon Telescope. It would combine the light from a worldwide constellation of radio telescopes. They’re hoping to actually image the event horizon of a black hole, and could have their first images within 5 years. Hopefully it’ll never get loaded onto a ship with Sam Neill.

Here’s hoping we’re just a few years away from knowing what black holes look like directly. But once seen, they can never be unseen. What do you think it’ll look like? tell us in the comments below!

And if you like what you see, come check out our Patreon page and find out how you can get these videos early while helping us bring you more great content!

September 24, 2014December 23, 2015 by Brian Koberlein

New Results from Planck: It Doesn’t Look Good For BICEP2

Dust map of the Universe. The region studied by BICEP2 is indicated by the rectangle in the right circle. Credit: Planck Collaboration

One of the recent sagas in cosmology began with the BICEP2 press conference announcing evidence of early cosmic inflation. There was some controversy since the press release was held before the paper was peer reviewed. The results were eventually published in Physical Review Letters, though with a more cautious conclusion than the original press release. Now the Planck team has released more of their data. This new work hasn’t yet been peer reviewed, but it doesn’t look good for BICEP2.

As you might recall, BICEP2 analyzed light from the cosmic microwave background (CMB) looking for a type of pattern known as B-mode polarization. This is a pattern of polarized light that (theoretically) is caused by gravitational waves produced by early cosmic inflation. There’s absolutely no doubt that BICEP2 detected B-mode polarization, but that’s only half the challenge. The other half is proving that the B-mode polarization they saw was due to cosmic inflation, and not due to some other process, mainly dust. And therein lies the problem. Dust is fairly common in the Milky Way, and it can also create B-mode polarization. Because the dust is between us and the CMB, it can contaminate its B-mode signal. This is sometimes referred to as the foreground problem. To really prove you have evidence of B-mode polarization in the CMB, you must ensure that you’ve eliminated any foreground effects from your data.

When the BICEP2 results were first announced, the question of dust was immediately raised. Some researchers noted that dust particles caught in magnetic fields could produce stronger B-mode effects than originally thought. Others pointed out that part of the data BICEP2 used to distinguish foreground dust wasn’t very accurate. This is part of the reason the final results went from “We found inflation!” to “We think we’ve found inflation! (But we can’t be certain.)”

Dust effects seen by Planck (shaded region) compared with inflation results of BICEP2 (solid line). Credit: Planck Collaboration — Dust effects seen by Planck (shaded region) compared with inflation results of BICEP2 (solid line).
Credit: Planck Collaboration

The new results from Planck chip at that claim even further. Whereas BICEP2 looked at a specific region of the sky, Planck has been gathering data across the entire sky. This means lots more data that can be used to distinguish foreground dust from a CMB signal. This new paper presented a map of the foreground dust, and a good summary can be seen in the figure. The shaded areas represents the B-mode levels due to dust at different scales. The solid line represents the B-mode distribution due to inflation as seen by BICEP2. As you can see, it matches the dust signal really well.

The simple conclusion is that the results of BICEP2 have been shown to be dust, but that isn’t quite accurate. It is possible that BICEP2 has found a mixture of dust and inflation signals, and with a better removal of foreground effects there may still be a real result. It is also possible that it’s all dust.

While this seems like bad news, it actually answers a mystery in the BICEP2 results. The level of inflation claimed by BICEP2 was actually quite large. Much larger than expected than many popular models. The fact that a good chuck of the B-mode polarization is due to dust means that inflation can’t be that large. So small inflation models are back in favor. It should also be emphasized that even if the BICEP2 results are shown to be entirely due to dust, that doesn’t mean inflation doesn’t exist. It would simply mean we have no evidence either way.

It’s tempting to look at all this with a bit of schadenfreude. Har, har, the scientists got it wrong again. But a more accurate view would be of two rival sports teams playing an excellent game. BICEP2 almost scored, but Planck rallied an excellent defense. Both teams want to be the first to score, but the other team won’t let them cheat to win. And we get to watch it happen.

Anyone who says science is boring hasn’t been paying attention.

Here’s the paper from the Planck team.

September 5, 2014December 23, 2015 by Brian Koberlein

What Would It Be Like To Fall Into A Black Hole?

Let’s say you happened to fall into the nearest black hole? What would you experience and see? And what would the rest of the Universe see as this was happening?

Let’s say you decided to ignore some of my previous advice. You’ve just purchased yourself a space dragon from the Market on the Centauri Ringworld, strapped on your favorite chainmail codpiece and sonic sword and now you’re going ride head first into the nearest black hole.

We know it won’t take you to another world or galaxy, but what would you experience and see on your way to your inevitable demise? And what would the rest of the Universe see as this was happening, and would they point and say “eewwwwww”?

If you were falling toward a black hole, most of the time you would simply feel weightless, just as if you were playing Bowie songs and floating in a most peculiar way in the International Space Station. The gravity of a black hole is just like the gravity of any other large mass, as long as you don’t get too close. But, as we’ve agreed, you’re ignoring my advice and flying dragon first into this physics nightmare. As you get closer, the gravitational forces on various parts of your and your dragon’s body would be different. Technically this is always true, but you wouldn’t notice it… at least at first.

Suppose you were falling feet first toward a black hole. As you got closer, your feet would feel a stronger force than your head, for example. These differences in forces are called tidal forces. Because of the tidal forces it would feel as if you are being stretched head to toe, while your sides would feel like they are being pushed inward. Eventually the tidal forces would become so strong that they would rip you apart. This effect of tidal stretching is sometimes boringly referred to as spaghettification.

I’ve made up some other names for it, such as My Own Private String Cheese Incident, “the soft-serve effect” and “AAAHHHHH AHHHH MY LEGS MY LEGS!!!”.

So, let’s summarize. You wouldn’t survive falling toward a black hole because you wouldn’t listen. Why won’t you ever listen?

A friend watching you fall toward a black hole would never see you reach the black hole. As you fall towards it, gravity would cause any light coming from you to be redshifted. So as you approached the black hole you would appear more and more reddish, and your image would appear dimmer and dimmer. Your friend would see you redden and dim as you approach, but never quite reach, the event horizon of the black hole. If they could still see you past this point, there would be additional red from the inside of you clouding up the view.

Artist's conception of the event horizon of a black hole. Credit: Victor de Schwanberg/Science Photo Library — Artist’s conception of the event horizon of a black hole. Credit: Victor de Schwanberg/Science Photo Library

Hypothetically, if you could survive crossing the event horizon of a black hole, what
would you see then? Contrary to popular belief, you would not see the entire future of the universe flash before you.

What you would see is the darkness of the black hole fill your view and as you approached the event horizon you would see stars and galaxies on the edge of your view being gravitationally lensed by the black hole. The sky would simply appear more and more black until you reach the event horizon.

Many people think that it is at the event horizon where you would be ripped apart, and at the event horizon all sorts of strange things occur. Unfortunately, this goes along with those who suspect black holes are actually some sort of portal. For a solar mass black hole, the tidal forces near the event horizon can be quite large, but for a supermassive black hole they aren’t very large at all.

In fact, the larger the black hole, the weaker the tidal forces near its event horizon. So if you happened to be near a supermassive black hole, you could cross the event horizon without really noticing. Would you still be totally screwed? YOU BETCHA!

What do you think? If you could drop anything into a black hole, what would it be? Tell us in the comments below.

August 11, 2014December 23, 2015 by Brian Koberlein

How Big is the Universe?

Hubble infrared image showing CL J1449+0856, the most distant mature cluster of galaxies found. Color data was added from ESO’s Very Large Telescope and the NAOJ’s Subaru Telescope. Credit: NASA, ESA, R. Gobat (Laboratoire AIM-Paris-Saclay, CEA/DSM-CNRS–)

The Universe is big, but how big is it? That all depends on whether the Universe is finite or infinite. Even the word “big” is tough to get clear. Are we talking about the size of the Universe we can see, or the Universe’s actual size right now?

The Universe is big, but how big is it? And what the heck kind of question is that? Are elephants big? Trucks? Dinosaurs? Cheese? Is cheese big? How big is cheese? How big is big?

The word “big” is tough to get clear. Are we talking about the size of the Universe we can see, or the Universe’s actual size right now? This becomes even more complicated when we are trying to work under assumptions of either the Universe is finite or the Universe is infinite.

One difficulty with talking about the size, is that the Universe is expanding. Light takes time to travel from distant galaxies, and while that light travels, the Universe continues to expand. So our problem with talking about how big it is, is that there is no single meaning to distance when it comes to the universe. For this reason, astronomers usually don’t worry about the distance to galaxies at all, and instead focus on redshift, which is measured by z. The bigger the z, the more redshift, and the more distant the galaxy.

As an example, consider one of the most distant galaxies we’ve observed, which has a redshift of 7.5. Using this, we can determine distance by calculating how long the light has traveled to reach us. With a redshift of 7.5, that comes out to be about 13 billion years. You might think that means it’s 13 billion light years away, but 13 billion years ago the universe was smaller, so it was actually closer at the time the light left that galaxy. Using this, if you calculate that distance, it was only a short 3.4 billion light years away.

Now the galaxy is much farther than that. After the light left the galaxy, the galaxy continued to move away from us. It is now about 29 billion light years away. Which is definitely more than 13, and quite a bit more than its original 3.4.

Usually it is this big distance that people mean when they ask for the size of the universe. This is known as the co-moving distance. Of course, we can only see so far. So, how far can we see? The most distant light we are able to observe is from the cosmic microwave background, which has a redshift of about z = 1,000.

This means the co-moving distance of the cosmic background is about 46 billion light years. Sticking us at the center of a massive sphere, the currently observable universe has a diameter of about 92 billion light years. Even with this observed distance, we know that it extends much further than that. If what we could see was all there is, we would see galaxies tend to gravitate towards us, which we don’t observe.

Multiverse Theory — Artist concept of the multiverse. Credit: Florida State University

In fact we don’t see any kind of galaxy clumping to a particular point at all. So as far as we know the universe could extend forever. It could be even stranger than that. Despite some media controversy, if the BICEP2 detection of early inflation is correct, it is likely the Universe undergoes a type of inflation with the intimidating moniker of “eternal inflation”. If it is the case, our observable universe is merely one bubble within an endless sea of other bubble universes. This is otherwise referred to as… the multiverse.

So, in the immortal words of Douglas Adams, “Space,” it says, “is big. Really big. You just won’t believe how vastly, hugely, mindbogglingly big it is. I mean, you may think it’s a long way down the road to the chemist’s, but that’s just peanuts to space”

What do you think? Does the Universe go on for ever? Tell us in the comments below. And if you like what you see, come check out our Patreon page and find out how you can get these videos early while helping us bring you more great content!

July 31, 2014December 23, 2015 by Brian Koberlein

What Are Comet Tails?

The view of Comet PANSTARRS L4 on 03-22-2013 over Warrenton, Virginia. Modified Canon Rebel Xsi DSLR 30 second exposure, ISO 1600, University Optics 80mm F6 Refractor (600mm). Credit and copyright: John Chumack.

Comets are renowned for their big beautiful tails that stretch across the sky. But what’s in those things, anyway? And how can comets get multiple tails?

In the past, humans generally used one of two greetings for comets:
1. Dear God, what is that thing? Terrible omens! Surely we will all die in fire.
2. Dear God, what is that thing? Great omens! Surely we will all have a big party… where we all die in fire?

For example, the appearance of what came to be known as Halley’s comet in 1066 was seen as a bad omen for King Harold II. Conversely, it was a good omen for William the Conqueror.

Because of their tails and transitory nature, comets were long thought to be products of the Earth’s atmosphere. It wasn’t until the 1500s, when Tycho Brahe used parallax to determine a comet’s distance. He realized that they were Solar System objects, like planets.

So, good news, we no longer regard them as omens and everyone stopped panicking. Right? Wrong. When Comet Halley approached Earth in 1910, astronomers detected cyanide gas in its tail. French astronomer Camille Flammarion was quoted as saying the gas could “impregnate the atmosphere and possibly snuff out all life on the planet.” This caused a great deal of hysteria. Many bought gas masks and “comet pills” to protect themselves.

With the rise of photographic astronomy, it was found that comets often have two types of tails. A bright tail composed of ionized gas, and a dimmer one composed of dust particles. The ion tail always points away from the Sun. It’s actually being pushed away from the comet by the solar wind.

Comets often develop two tails as they near the sun - a curved dust tail and straight, ion tail. Credit: NASA — Comets often develop two tails as they near the sun – a curved dust tail and straight, ion tail. Credit: NASA

We now know that a comet’s ion tail contains “volatiles” such as water, methane, ammonia and carbon dioxide. These volatiles are frozen near the comet’s surface, and as they approach the Sun, they warm and become gaseous. This also causes dust on the comet’s surface to stream away. The heating of a comet by the Sun is not uniform.

Because of a comet’s irregular shape and rotation, some parts of the surface can be heated by sunlight, while other parts remain cold. In some cases this can mean that comets can have multiple tails, which creates amazing effects where different regions of a comet stream off volatiles.

Comet Lovejoy passing behind green oxygen and sodium airglow layers on December 22, 2011 seen from the space station. Credit: NASA/Dan Burbank

These ion tails can be quite large, and some have been observed to be nearly 4 times the distance of the Earth from the Sun. And even though they fill a great volume, they are also pretty diffuse. If you condensed a comet’s tail down to the density of water, it wouldn’t even fill a swimming pool.

We also now know that there isn’t a clear dividing line between comets and asteroids. It’s not the case the comets are dirty snowballs and asteroids are dry rocks. There is a range of variation, and asteroids can gain dusty or gaseous tails and take on a comet-like appearance. In addition, we’ve also found comets orbiting other stars, known as exocomets.

And finally one last fact, the term comet comes from the Latin cometa, which indicated a hairy star.

So, what’s your favorite comet? Tell us in the comets below. And if you like what you see, come check out our Patreon page and find out how you can get these videos early while helping us bring you more great content!

July 17, 2014December 23, 2015 by Brian Koberlein

Is Our Solar System Weird?

This artist’s view shows an extrasolar planet orbiting a star (the white spot in the right). Image Credit: IAU/M. Kornmesser/N. Risinger (skysurvey.org)

Is our Solar System normal? Or is it weird? How does the Solar System fit within the strange star systems we’ve discovered in the Milky Way so far?

With all the beautiful images that come down the pipe from Hubble, our Solar System has been left with celestial body image questions rivaling that of your average teenager. They’re questions we’re all familiar with. Is my posture crooked? Do I look pasty? Are my arms too long? Is it supposed to bulge out like this in the middle? Some of my larger asteroids are slightly asymmetrical. Can everyone tell? And of course the toughest question of all… Am I normal?

The idea that stars are suns with planets orbiting them dates back to early human history. This was generally accompanied by the idea that other planetary systems would be much like our own. It’s only in the last few decades that we’ve had real evidence of planets around other stars, known as exoplanets. The first extrasolar planet was discovered around a pulsar in 1992 and the first “hot jupiter” was discovered in 1995.

Most of the known exoplanets have been discovered by the amazing Kepler spacecraft. Kepler uses the transit method, observing stars over long periods of time to see if they dim as a planet passes in front of the star. Since then, astronomers have found more than 1700 exoplanets, and 460 stars are known to have multiple planets. Most of these stellar systems are around main sequence stars, just like the Sun. Leaving us with plenty of systems for comparison.

Artist's impression of the solar system showing the inner planets (Mercury to Mars), the outer planets (Jupiter to Neptune) and beyond. Credit: NASA — Artist’s impression of the solar system showing the inner planets (Mercury to Mars), the outer planets (Jupiter to Neptune) and beyond. Credit: NASA

So, is our Solar System normal? Planets in a stellar system tend to have roughly circular orbits, just like our Solar system. They have a range of larger and smaller planets, just like ours. Most of the known systems are even around G-type stars. Just like ours.….and we are even starting to find Earth-size planets in the habitable zones of their stars. JUST LIKE OURS!

Not so fast…Other stellar systems don’t seem to have the division of small rocky planets closer to the star and larger gas planets farther away. In fact, large Jupiter-type planets are generally found close to the star. This makes our solar system rather unusual.

Computer simulations of early planetary formation shows that large planets tend to move inward toward their star as they form, due to its interaction with the material of the protoplanetary disk. This would imply that large planets are often close to the star, which is what we observe. Large planets in our own system are unusually distant from the Sun because of a gravitational dance between Jupiter and Saturn that happened when our Solar System was young.

Although our Solar System is slightly unusual, there are some planetary systems that are downright quirky. There are planetary systems where the orbits are tilted at radically different angles, like Kepler 56, and a sci-fi favorite, the planets that orbit two stars like Kepler 16 and 34. There is even a planet so close to its star that its year lasts only 18 hours, known 55 Cancri e.

And so, the Kepler telescope has presented us with a wealth of exoplanets, that we can compare our beautiful Solar System to. Future telescopes such as Gaia, which was launched in 2013, TESS and PLATO slated for launch in 2017 and 2024 will likely discover even more. Perhaps even discovering the holy grail of exoplanets, a habitable planet with life…

And the who knows, maybe we’ll find another planet… just like ours.

What say you? Where should we go looking for habitable worlds in this big bad universe of ours? Tell us in the comments.

And if you like what you see, come check out our Patreon page and find out how you can get these videos early while helping us bring you more great content!

July 14, 2014December 23, 2015 by Brian Koberlein

What Is The Great Attractor?

What is at the Center of the Milky Way — Examining the Center of the Milky Way

There’s a strange place in the sky where everything is attracted. And unfortunately, it’s on the other side of the Milky Way, so we can’t see it. What could be doing all this attracting?

Just where the heck are we going? We’re snuggled in our little Solar System, hurtling through the cosmos at a blindingly fast of 2.2 million kilometers per hour. We’re always orbiting this, and drifting through that, and it’s somewhere out in the region that’s not as horrifically terrifying as what some of our celestial neighbors go through. But where are we going? Just around in a great big circle? Or an ellipse? Which is going around in another circle… and it’s great big circles all the way up?

Not exactly… Our galaxy and other nearby galaxies are being pulled toward a specific region of space. It’s about 150 million light years away, and here is the best part. We’re not exactly sure what it is. We call it the Great Attractor.

Part of the reason the Great Attractor is so mysterious is that it happens to lie in a direction of the sky known as the “Zone of Avoidance”. This is in the general direction of the center of our galaxy, where there is so much gas and dust that we can’t see very far in the visible spectrum. We can see how our galaxy and other nearby galaxies are moving toward the great attractor, so something must be causing things to go in that direction. That means either there must be something massive over there, or it’s due to something even more strange and fantastic.

When evidence of the Great Attractor was first discovered in the 1970s, we had no way to see through the Zone of Avoidance. But while that region blocks much of the visible light from beyond, the gas and dust doesn’t block as much infrared and x-ray light. As x-ray astronomy became more powerful, we could start to see objects within that region. What we found was a large supercluster of galaxies in the area of the Great Attractor, known as the Norma Cluster. It has a mass of about 1,000 trillion Suns. That’s thousands of galaxies.

A March 2013 picture of the Shapley Supercluster from the European Space Agency's Planck observatory. ESA describes it as "the largest cosmic structure in the local Universe." Credit: ESA & Planck Collaboration / Rosat/ Digitised Sky Survey — A March 2013 picture of the Shapley Supercluster from the European Space Agency’s Planck observatory. ESA describes it as “the largest cosmic structure in the local Universe.” Credit: ESA & Planck Collaboration / Rosat/ Digitised Sky Survey

While the Norma Cluster is massive, and local galaxies are moving toward it, it doesn’t explain the full motion of local galaxies. The mass of the Great Attractor isn’t large enough to account for the pull. When we look at an even larger region of galaxies, we find that the local galaxies and the Great Attractor are moving toward something even larger. It’s known as the Shapley Supercluster. It contains more than 8000 galaxies and has a mass of more than ten million billion Suns. The Shapley Supercluster is, in fact, the most massive galaxy cluster within a billion light years, and we and every galaxy in our corner of the Universe are moving toward it.

So as we hurtle through the cosmos, gravity shapes the path we travel. We’re pulled towards the Great Attractor, and despite its glorious title, it appears, in fact to be a perfectly normal collection of galaxies, which just happens to be hidden.

What do you think? What are you hoping we’ll discover over in the region of space we’re drifting towards?

And if you like what you see, come check out our Patreon page and find out how you can get these videos early while helping us bring you more great content!

May 19, 2014December 23, 2015 by Brian Koberlein

Are the BICEP2 Results Invalid? Probably Not.

Galactic radio loops, with BICEP2 region indicated. Credit: Philipp Mertsch

Recently rumors have been flying that the BICEP2 results regarding the cosmic inflationary period may be invalid. It all started with a post by Dan Falkowski on his blog Resonaances, where he claimed that the BICEP2 had misinterpreted some data, which rendered their results invalid, or at least questionable. The story was then picked up by Nature’s Blog and elsewhere, which has sparked some heated debate.

So what’s really going on?

For those who might not remember, BICEP2 is a project working to detect polarized light within the cosmic microwave background (CMB). Specifically they were looking for a type of polarization known as B-mode polarization. Detection of B-mode polarization is important because one mechanism for it is cosmic inflation in the early universe, which is exactly what BICEP2 claimed to have evidence of.

Part of the reason BICEP2 got so much press is because B-mode polarization is particularly difficult to detect. It is a small signal, and you have to filter through a great deal of observational data to be sure that your result is valid. But you also have to worry about other sources that look like B-mode polarization, and if you don’t account for them properly, then you could get a “false positive.” That’s where this latest drama arises.

In general this challenge is sometimes called the foreground problem. Basically, the cosmic microwave background is the most distant light we can observe. All the galaxies, dust, interstellar plasma and our own galaxy is between us and the CMB. So to make sure that the data you gather is really from the CMB, you have to account for all the stuff in the way (the foreground). We have ways of doing this, but it is difficult. The big challenge is to account for everything.

A map of foreground polarization from the Milky Way. Credit: ESA and the Planck Collaboration

Soon after the BICEP2 results, another team noted a foreground effect that could effect the BICEP2 results. It involves an effect known as radio loops, where dust particles trapped in interstellar magnetic fields can emit polarized light similar to B-mode polarization. How much of an effect this might have is unclear. Another project being done with the Planck satellite is also looking at this foreground effect, and has released some initial results (seen in the figure), but hasn’t yet released the actual data yet.

Now it has come to light that BICEP2 did, in fact, take some of this foreground polarization into account, in part using results from Planck. But since the raw data hadn’t been released, the team used data taken from a PDF slide of Planck results and basically reverse-engineered the Planck data. It is sometimes referred to as “data scraping”, and it isn’t ideal, but it works moderately well. Now there is some debate as to whether that slide presented the real foreground polarization or some averaged polarization. If it is the latter, then the BICEP2 results may have underestimated the foreground effect. Does this mean the BICEP2 results are completely invalid? Given what I’ve seen so far, I don’t think it does. Keep in mind that the Planck foreground is one of several foreground effects that BICEP2 did account for. It could be a large error, but it could also be a rather minor one.

The important thing to keep in mind is that the BICEP2 paper is still undergoing peer review. Critical analysis of the paper is exactly what should happen, and is happening. This type review used to be confined to the ivory towers, but with social media it now happens in the open. This is how science is done. BICEP2 has made a bold claim, and now everyone gets to whack at them like a piñata.

The BICEP2 team stands by their work, and so we’ll have to see whether it holds up to peer review. We’ll also have to wait for the Planck team to release their results on B-mode polarization. Eventually the dust will settle and we’ll have a much better handle on the results.