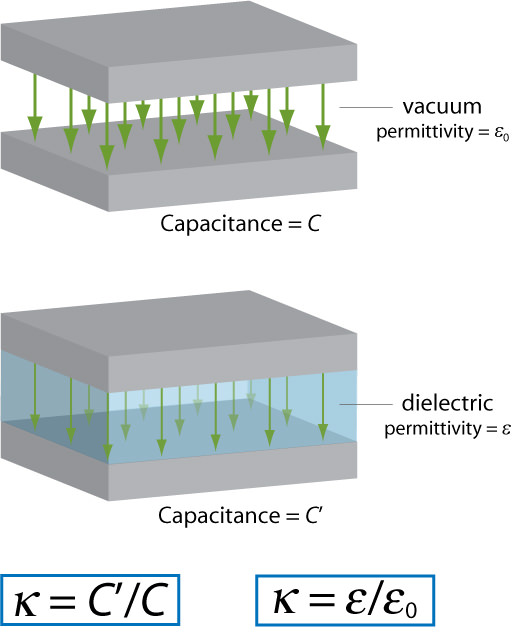
[/caption]Take a look at that ceramic toilette or sink in your bathroom. Ever think to yourself that it has something in common with glass, mica, plastic, or even dry air? Ever consider that it might be useful in the construction of capacitors. Probably not, but that’s because this material has a property that is often overlooked. It is a dielectric, meaning a substance that is a poor conductor of electricity, but a good means of electrical storage. Whether we are talking about ceramic, glass, air, or even vacuum (another good dielectric), scientists use what is called the Dielectric Constant, which is the ratio of permittivity of a substance to the permittivity of free space. Or, in layman’s terms, the ratio of the amount of electrical energy stored in a material by an applied voltage, relative to that stored in a vacuum.
Confused? Well, perhaps a little explanation is necessary to dispel some of the technical roadblocks to understanding. First of all, a dielectric is defined as an insulating material or a very poor conductor of electric current. When dielectrics are placed in an electric field, practically no current flows in them because, unlike metals, they have no loosely bound, or free, electrons that may drift through the material. Instead, electric polarization occurs, where the positive charges within the dielectric are displaced minutely in the direction of the electric field, and the negative charges are displaced minutely in the direction opposite to the electric field. This slight separation of charge, or polarization, reduces the electric field within the dielectric itself. This property, as already mentioned, makes it a poor conductor, but a good storage medium.
In practice, most dielectric materials are solid. But, as already mentioned, dry air is also dielectric, as are most pure, dry gases such as helium and nitrogen. These have a low dielectric constant, whereas things like metal oxides have a high constant. Materials with moderate dielectric constants include ceramics, distilled water, paper, mica, polyethylene, and glass. As the dielectric constant increases, the electric flux density increases (the total amount of electrical charge per area), but only if all other factors remain unchanged. This in turn enables objects of a given size, such as sets of metal plates, to hold their electric charge for long periods of time, and/or to hold large quantities of charge.
Because of they constitute good insulating material (or dielectric), metal oxides, dry air and vacuum are often used in the construction of high-energy capacitors as well as radio-frequency transmission lines, where electrical energy is stored at radio frequencies.
We have written many articles about the dielectric constant for Universe Today. Here’s an article about how microwaves work, and here’s an article about the table-top test of general relativity.
If you’d like more info on dielectric constant, check out these articles from Hyperphysics and Web Physics.
We’ve also recorded an entire episode of Astronomy Cast all about Electromagnetism. Listen here, Episode 103: Electromagnetism.
Sources:
http://en.wikipedia.org/wiki/Dielectric
http://en.wikipedia.org/wiki/Relative_permittivity
http://en.wikipedia.org/wiki/Flux
http://en.wikipedia.org/wiki/Electrostatic
http://www.britannica.com/EBchecked/topic/162637/dielectric-constant
http://searchcio-midmarket.techtarget.com/sDefinition/0,,sid183_gci546287,00.html
http://www.britannica.com/EBchecked/topic/162630/dielectric