Posted on by Jon Voisey

Measuring the Moon’s Eccentricity at Home

View of the moon at perigee and apogee

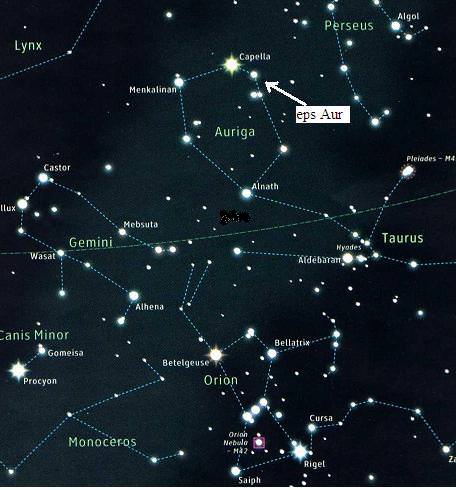
Caption: View of the moon at perigee and apogee

As a teacher, I’m always on the lookout for labs with simple setups appropriate for students. My current favorite is finding the speed of light with chocolate.

In a new paper recently uploaded to arXiv, Kevin Krisciunas from Texas A&M describes a method for determining the orbital eccentricity of the moon with a surprisingly low error using nothing more than a meter stick, a piece of cardboard and a program meant for fitting curves to variable stars.

This method makes use of the fact that the eccentricity can be determined from the ratio of the mean angular size of an object and one half of its amplitude. Thus, the main objective is to measure these two quantities.

Kevin’s strategy for doing this is to make use of a cardboard sighting hole which can slide along a meter stick. By peering through the hole at the moon, and sliding the card back and forth until the angular size of the hole just overlaps the moon. From there, the diameter of the hole divided by the distance down the meter stick gives the angular size thanks to the small angle formula (? = d/D in radians if D >> d).

To prevent systematic errors in misjudging as the card is slid forward until the size of the hole matches the moon, it is best to also approach it from the other direction; Coming from in from the far end of the meter stick. This should help reduce errors and in Kevin’s attempt, he found that he had a typical spread of ± 4 mm when doing so.

At this point, there is still another systematic error that must be taken into account: The pupil has a finite size comparable to the sighting hole. This will cause the actual angular size to be underestimated. As such, a correction factor is necessary.

To derive this correction factor, Kevin placed a 91 mm disk at a distance of 10 meters (this should produce a disk with the same angular size as the moon when viewed from that distance). To produce the best match, the slip of cardboard with the sighting hole should need to be placed at 681.3 mm on the meter stick, but due to the systematic error of the pupil, Kevin found it needed to be placed at 821 mm. The ratio of the observed placement to the proper placement provided the correction factor Kevin used (1.205). This would need to be calibrated for each individual person and would also depend on the amount of light during the time of observation since this also affects the diameter of the pupil. However, adopting a single correction factor produces satisfactory results.

This allows for properly taken data which can then be used to determine the necessary quantities (the mean angular size and 1/2 the amplitude). To determine these, Kevin used a program known as PERDET which is designed for fitting sinusoid curves to oscillations in variable stars. Any program that could fit such curves to data points using a ?2 fit or a Fourier analysis would be suitable to this end.

From such programs once the mean angular size and half amplitude are determined, their ratio provides the eccentricity. For Kevin’s experiment, he found a value of 0.039 ± 0.006. Additionally, the period he determined from perigee to perigee was 27.24 ± 0.29 days which is in excellent agreement with the accepted value of 27.55 days.

Posted on by Nancy Atkinson

Galaxy Zoo is Expanding to Include a Whole New “Zooniverse”

Galaxy Zoo has been an enormously successful citizen science project; so much so, that other astronomers, as well as scientists from other disciplines, have taken notice and now they want to get in on the act of having the public help make discoveries about our world and Universe. Today, the Galaxy Zoo team has launched Zooniverse. This new website will be a platform, or “home” to a plethora of new science projects where the public can take their pick of where and how they can make meaningful contributions and discoveries.

“Zooniverse became inevitable around the time that Galaxy Zoo launched in 2007,” said Chris Lintott, one of the founders of the original Galaxy Zoo, and now Zooniverse, “because it was obvious a few hours into the first day that we had hit on a way of doing science that was really powerful. And it was clear that this was not only going to work with for galaxies, but for other science as well. Soon we’ll we have solar investigations, climate science, and a lot of other citizen science projects coming online.”

For those “Zooites” who love Galaxy Zoo – don’t worry, that project isn’t going anywhere.

“Galaxy Zoo itself will remain the sort of comfortable old sofa in the corner,” Lintott told Universe Today, “so anyone who is comfortable with that can remain sitting there, classifying galaxies and discovering things. But for people who want to explore a bit further and find new and exciting places to be, we’re going to expand the Zooniverse by pointing some new data to the sofa.”

On Dec. 16 an astrophysics project will be introduced in Beta to people already involved in Galaxy Zoo and the offshoot projects: Galaxy Zoo 2, Supernova Hunt, and Galaxy Mergers. If all goes well, it will be launched “live” to the public early 2010.

With new advanced instruments and ways of gathering data, scientists in almost all disciplines are inundated with data but don’t have an efficient way to sort through, organize and classify the information. Galaxy Zoo’s success (over 51 million classifications by over 250,000 people, as well as new discoveries and several science papers published) has attracted the attention of other scientists, many who have contacted Lintott and his team, wondering if there was any way they could use that same model to have the public help with other unique science tasks.

Lintott said they have a long list of additional projects that are already under development. “In a couple of years’ time we should have some wonderful projects come online, such as studying ancient artifacts, oceanography projects, looking at Earth from space, animal behavior projects, and more. We keep getting new really great projects contacting us all the time.”

“We’ve known this has been coming for years, but we didn’t really know how to do it,” said Arfon Smith from Oxford University, one of the developers of the Zooniverse site, who has been working on how to integrate other science projects into the Galaxy Zoo model. “We needed a big project to come along that wasn’t dealing with galaxies to actually get us pointed in the right direction. The technical challenge was to make the Zooniverse a nice place to be, and to make it easy for users to move between the different ‘Zoos.'”

If you are registered on Galaxy Zoo, you’ll notice you can now access and seamlessly move between Galaxy Zoo, the Mergers and Supernova Hunt sites without re-logging in. The same will hold true for the new science projects that will be coming online on the Zooniverse in the coming months and years.

“There’s an opportunity here for people to explore a range of citizen science projects,” said Smith. “Zooniverse will be a place where people can check to see what projects they might want to work on.”

“The common thread is that each project needs the public’s help to increase our understanding of the Universe, and each will produce results that could not happen without the public,” said Lintott. “Soon, there should be a science project for everyone’s interests.”

Lintott said Universe Today readers will have to wait a little while to see what they actually are, but we will definitely keep everyone updated on the new citizen science projects as they become available.

Alongside the Zooniverse, another new website, Citizen Science Alliance, has been launched for the organizations who will be coming to the Zooniverse. “The Citizen Science Alliance involves our partners,” said Lintott, “and all of us believe that making use the public’s skills, talents and energy is not only helpful in dealing with the flood of data confronting us, but it is necessary.”

Source: interview with Chris Lintott and Arfon Smith

Posted on by Nancy Atkinson

Reaching Near Space For Less Than $150

Earth from 93,000 feet. Long Island in the background. Credit: The 1337Arts Group


A group of MIT students have launched a low-budget satellite to near space, taking images of the curvature of Earth and the blackness of space. Their approach was to use low tech, off the shelf equipment, which included a Styrofoam beer cooler, a camera from eBay, open source software and an inexpensive helium balloon as the launch vehicle in order to do their complete mission launch for less than $150. Total cost? $148. The experience? Priceless, including getting interviewed on CNN and Fox News about their achievement. The best news for the rest of us? They’ll soon be sharing an illustrated step-by-step guide on how to launch your own low-budget satellite.

The team, led by Justin Lee and Oliver Yeh had the goal of seeing Earth from space, but didn’t have a lot of money to do it. They knew they’d have to gather all the materials for less than $150.
[/caption]
Their satellite was a huge success. It reached 93,000 feet (calculated from the linear ascent rate at the beginning of the launch), took several images of Earth from space (see their gallery here) and was retrieved using an inexpensive GPS system.

They say the time lapse video above isn’t all that great because the cooler wasn’t stabilized. But the images are incredible.

Many people have launched balloons (see some of our previous articles, here and here) but this is the lowest price to space anyone has ever accomplished. The students say they hope to be an inspiration to others.

The balloon falling back to Earth after bursting. Credit: 1337arts team.
The balloon falling back to Earth after bursting. Credit: 1337arts team.

Lee and Yeh caution about making sure future explorers contact the FAA about launching a balloon, and to launch from a safe place so the balloon and equipment doesn’t land in a highly populated area.

Next, they want to do it again, but add a rocket to the balloon to launch their payload even higher.

Check out their website for more info and the great images.

Posted on by Nancy Atkinson

Create Your Own Galaxy Mashup With New Galaxy Zoo Tool

M81. Image credit: NASA/JPL-Caltech/ESA/Harvard-Smithsonian CfA

[/caption]
If you haven’t yet succumbed to the temptation of Galaxy Zoo, a new add-on to the popular citizen scientist project just might catapult you into joining the thousands of people who are clicking and classifying. Galaxy Zoo has now teamed up with Microsoft’s World Wide Telescope to allow users to immerse themselves in the universe and be able to easily create videos and sky tours that can be customized and shared with friends and family. “Now there is an easy way to inflict your favorites on others,” said Galaxy Zoo team member Dr. Pamela Gay.

The new Sky Tour tool, available here was created by two of Gay’s students at Southern Illinois University Edwardsville, sophomores Jarod Luebbert and Mark Sands.

On Galaxy Zoo, the Zooites work with isolated images of galaxies to classify them by shape and other features. Coordinating with WWT allows users to see the galaxies in their home environments on the sky. “It’s so easy to classify a few hundred — or even a few thousand galaxies and think you’ve seen a reasonable chunk in the sky,” Gay told Universe Today. “But then you start looking at them in WWT and realize each galaxy is just a pinhead of light in a vast, vast sky. Jarod and Mark’s work really gives us a since of scale and how small we all are.”

To give you a taste of how this interface works, Luebbert and Sands created a great teaser video.

Galaxy Zoo – WorldWide Telescope Mashup! from Galaxy Zoo on Vimeo.

(The music on the video is great! Even though the video says “Starts Tomorrow,” tomorrow has now arrived, and the Sky Tour tool is available to use.)

GZ users need to classify at least 100 galaxies before the Sky Tour tool works with their “favorites.”

Tours can be created and customized with music, pictures, and logos. Other new features include sharing directly to networking sites, and competition with other Galaxy Zoo users.

But how do college students get a chance to work on a project with Microsoft and world class astronomers?

“We knew the job opportunity had become available that they wanted two teammates who would work well together for an excellent and educational project dealing with GalaxyZoo,” Sands told Universe Today. “Jarod and I being close friends, were encouraged to apply for this position by a fellow Zoo team member, Scott Miller. After being hired, we were accepted by the rest of the team and got right to work.”

Gay and Galaxy Zoo founder Chris Lintott presented the two students with a proposal they had sent to Microsoft explaining a very detailed approach to integrating Microsoft WorldWide Telescope with GalaxyZoo.

“The synopsis was simple, and we were to merge the creation of WorldWide Telescope tours with GalaxyZoo user favorites,” Sands said, “as well as implement a WordPress (a popular blogging software) plugin for educators to create WWT tours for podcasts (a project to be released in December). With no strict direction, Pamela allowed us to go wild and be creative with our own ideas.”

The two students began formulating ideas and creating dozens of mockups. Then in July, Sands and Luebbert found themselves arriving at Microsoft Research Building 99 in Redmond, WA collaborating directly with the architects of WorldWide Telescope.

Sands said WWT architect Jonathan Fay and Peter Turcan were readily available to help with the Galaxy Zoo project and were extremely helpful, as well as Kim Rush. Yan Xu from Microsoft worked directly with Gay and Lintott on the GalaxyZoo proposal.

“It was a blast to work with them and they helped us out a lot,” said Luebbert. “Even though the original idea came from Pamela and Chris, Mark and I added our own touches as we went along.”

“Working with Microsoft was an unimaginable experience,” Sands said. “There are some fantastic people who work there and deserve just as much attention as we do. I speak for both of us when I say we had a lot of fun working with them, even if it only lasted two weeks.”

The GZ/WWT integration has received great reviews from the users. “The ability of Galaxy Zoo’s volunteers to find interesting objects never ceases to amaze me,” said Lintott. “I’m looking forward to sitting back and enjoying their tours of the Universe.”

The citizen scientists of Galaxy Zoo have classified more than 100 million classifications galaxies since its launch in July 2007. Additionally, results from users have inspired more than 15 scientific papers to date.

Posted on by Nancy Atkinson

Help Solve the Mystery of Epsilon Aurigae with Citizen Sky

ESO Online Digitized Sky Survey

[/caption]
We’ve written about Epsilon Aurigae before, but this mysterious star is just now beginning to dim, so we wanted to remind everyone that they can be involved in real science and help solve a mystery! The variable star Epsilon Aurigae is now beginning its puzzling transformation that happens every 27 years. “That means the last time Epsilon Aurigae had an eclipse we were all rockin’ big hair and sporting shoulder pads in all of our clothes,” said Rebecca Turner, coordinator for a special project for the IYA organized by the American Association of Variable Star Observers (AAVSO). Astronomers can’t figure out why this mysterious star dims on a regular basis, so to help solve the mystery they are calling for assistance from thousands of citizen scientists.

That means you can help contribute to real astronomical research!

Since its discovery in 1821, the supergiant star Epsilon Aurigae has dipped in brightness like clockwork every 27.1 years as it is eclipsed by a very large companion object. But based on the shape of the lightcurve and the spectra that have taken of the system, astronomers can’t figure out what exactly what kind of object is eclipsing the star. Another strange feature of the lightcurve is that there is a slight brightening in the middle of the eclipse.

“The leading theory is that the secondary is surrounded by a large opaque disk,” said Turner, on the July 7 episode of the 365 Days of Astronomy podcast. “This would explain why light from the secondary doesn’t seem to be showing up in spectra. The disk seems to have a hole in the center, which would account for the mid-eclipse brightening. Current thinking is that perhaps the center of the disk is home to 2 less luminous, tightly orbiting stars. This tight orbit could create what astronomers are calling a gravitational eggbeater effect – creating that hole in the disk. Theories of a large planet falling into the stars at the center of the disk have also been introduced recently.”

Sky map of Epsilon Aurigae
Sky map of Epsilon Aurigae

Epsilon Aurigae is a bright star that can be seen with the unaided eye even in bright urban areas of the Northern Hemisphere from fall to spring. But it is also too bright for most professional telescopes to observe, so this is where the public comes in.

“It’s not just amateurs with fancy telescopes and CCDs or photoelectric photometers that are needed for this experiment,” said AAVSO’s Mike Simonsen. “People with just their eyes or a pair of binoculars can contribute to understanding this weird star by observing epsilon Aurigae over the next two years and reporting their observations to AAVSO.”

A diagram of the most popular model of the epsilon Aurigae system, by Jeff Hopkins:
A diagram of the most popular model of the epsilon Aurigae system, by Jeff Hopkins:

For this project, a new website has been launched called “Citizen Sky”, and all you need are a good pair of eyes, and a finder chart, which can be found on the website. No previous astronomical experience is necessary.

The project is supported by a three-year grant from the National Science Foundation to recruit, train, and coordinate public participation in this project. What makes this project different from previous citizen science projects is its emphasis on participation in the full scientific method. Participants are not being asked simply to collect data. They will also be trained to analyze data, create and test their own hypotheses, and to write papers for publication in professional astronomy journals. Participants can work alone on all phases of the project or they can focus on one stage and team up with others.

Epsilon Aurigae is just now beginning to dim. It will remain faint during all of 2010 before slowly regaining its normal brightness by the summer of 2011.

The lead astronomer for this project is Dr. Robert Stencel, the William Herschel Womble Professor of Astronomy at Denver University. Dr. Bob, as the amateur astronomy community knows him, studied the last event in 1982-84 while working at NASA. “This is truly an amazing star system. It contains both a supergiant star and a mysterious companion. If the supergiant was in our solar system, its diameter would extend to Earth, engulfing us,” Stencel said. “The companion only makes its presence known every 27 years and is a type of ‘dark matter’ in that we indirectly detect its presence but don’t know what it is.

“To make things even more fun, we also have some evidence of a substantial mass, perhaps a large planet, spiraling into the mysterious dark companion object. Observations during the upcoming eclipse will be key to understanding this and predicting what will happen if the putative planet does eventually fall into the star,” Dr. Bob added.

Here’s a video with Rebecca Turner explaining more about Citizen Sky.

For more on Epsilon Aurigae, see this page from AAVSO
Citizen Sky

Sources: 365 Days of Astronomy,

Posted on by Nancy Atkinson

Hunt for Supernovae With Galaxy Zoo

How would you like to find a supernova? I can’t think of anyone who wouldn’t be proud to say they have spotted an exploding star. And now, perhaps you can – and without all the work of setting up your telescope and staying up all night (well, that can be fun, too, but…). The great folks who brought you Galaxy Zoo have now partnered with the Palomar Transient Factory to offer the public a chance to hunt and click for supernovae from the comfort of your own computer. And yes, you can still classify galaxies at Galaxy Zoo, but now you can search for for the big guns out in space, too. Sound like fun?

The Palomar Transient Facory uses the famous Palomar Observatory and the Samuel Oschin 1.2 m telescope to look for anything that’s changing in the sky — whether it’s a variable star, an asteroid moving across the sky, the flickering of an active galaxy’s nucleus or a supernova. For now, though, the partnership with Galaxy Zoo will concentrate on finding supernovae, and in particular Type 1A supernovae.

According to Scott Kardel of the Palomar Observatory, “the quantity and quality of the new data that’s been coming in are absolutely mind blowing for astronomers working in this field. On one recent night PTF patrolled a section of the sky about five times the size of the Big Dipper and found eleven new objects.” For the supernova search, it returns to the same galaxies twice a night, every five nights.

That’s where the Zooites from Galaxy Zoo come in: searching through all specially chosen PTF data and looking for supernovae.

“Your task is to search through the candidates found by PTF” said the Galaxy Zoo team. “Waiting for your results are two intrepid Oxford astronomers, Mark and Sarah, who have travelled out to the Roque de los Muchachos Observatory on the Canary Island of La Palma. They have time allocated on the 4.2m William Herschel Telescope to follow up the best of our discoveries.”

Check out Galaxy Zoo’s Supernova page for more info and to sign up to be part of this exciting new Citizen Science project!

For more info on the Palomar Transient Factory, listen to Scott Kardel’s 365 Days of Astronomy podcast.

Posted on by Anne Minard

Galaxy Zoo Discovers New Group of Galaxies: ‘Green Peas’

The newly discovered Green Pea galaxies. (Photo: Carolin Cardamone and Sloan Digital Sky Survey.)

[/caption]

Citizen scientists from the Galaxy Zoo project have discovered rare galaxies they’re calling the “Green Peas.” They’re small in size, bright green in color, and proficient at churning out new stars — plus, they could reveal unique insights into how galaxies form stars in the early universe.

The newly discovered galaxies appear in the image at left, from Carolin Cardamone and the Sloan Digital Sky Survey.

“These are among the most extremely active star-forming galaxies we’ve ever found,” said Cardamone, an astronomy graduate student at Yale University and lead author of a new paper on the discovery. The results will appear in an upcoming issue of the Monthly Notices of the Royal Astronomical Society.

Galaxy Zoo users volunteer their spare time to help classify galaxies in an online image bank. Cardamone said of the one million galaxies that make up Galaxy Zoo’s image bank, the team found only 250 Green Peas.

“No one person could have done this on their own,” she said. “Even if we had managed to look through 10,000 of these images, we would have only come across a few Green Peas and wouldn’t have recognized them as a unique class of galaxies.”

The Green Peas boast “some of the highest specific star formation rates seen in the local Universe,” write Cardamone and her co-authors, “yielding doubling times for their stellar mass of hundreds of millions of years.”

The authors say evidence points to recent or ongoing mergers, adding that the Peas are similar in size, mass, luminosity and metallicity to Luminous Blue Compact Galaxies.

“They are also similar to high redshift UV-luminous galaxies, e.g., Lyman-break galaxies and Lyman-alpha emitters, and therefore provide a local laboratory with which to study the extreme star formation processes that occur in high-redshift galaxies,” they write.

The galaxies, which are between 1.5 billion and 5 billion light years away, are 10 times smaller than our own Milky Way galaxy and 100 times less massive. But they are forming stars 10 times faster than the Milky Way.

Kevin Schawinski, a postdoctoral associate at Yale and one of Galaxy Zoo’s founders, said the Green Peas would have been normal in the early universe, “but we just don’t see such active galaxies today. Understanding the Green Peas may tell us something about how stars were formed in the early universe and how galaxies evolve.”

The Galaxy Zoo volunteers who discovered the Green Peas—and who call themselves the “Peas Corps” and the “Peas Brigade”—began discussing the strange objects in the online forum. (The original forum thread was called “Give peas a chance.”)

Cardamone asked the volunteers, many of whom had no previous astronomy background or experience, to refine the sample of objects they detected in order to determine which were bona fide Green Peas and which were not, based on their colors. By analyzing their light, Cardamone determined how much star formation is taking place within the galaxies.

“This is a genuine citizen science project, where the users were directly involved in the analysis,” Schawinski said, adding that 10 Galaxy Zoo volunteers are acknowledged in the paper as having made a particularly significant contribution. “It’s a great example of how a new way of doing science produced a result that wouldn’t have been possible otherwise.”

Source: Yale University, via the American Astronomical Society press wire. The paper is here, and here is a link to the Galaxy Zoo project.

Posted on by Nancy Atkinson

Citizen Science: Help Solve the Mystery of Epsilon Aurigae

Auriga. Credit: Sky and Telescope

[/caption]
Epsilon Aurigae is a mystery. This variable star changes in brightness over time, and is thought to be an eclipsing binary. Some things about the way that this star fades and then regains it brightness are still not fully understood by astronomers, even after over 175 years of study. But now, you can help. The next eclipse of this star is predicted to begin in August 2009. Citizen Sky is a citizen science project providing you with a chance to do real scientific research to help solve the mystery.

Epsilon Aurigae is a very bright star — a third-magnitude F-type supergiant star — located in the constellation Auriga, the charioteer. This star is bright enough to be seen with the unaided eye even in the most light-polluted cities, and it is visible every autumn, winter, and spring. Because of its brightness, it can be observed by almost anyone regardless of background, training, or equipment. All you need are a good pair of eyes and know where to look.

Epsilon Aurigae model. Credit: NOAO, AURA, & NSF.
Epsilon Aurigae model. Credit: NOAO, AURA, & NSF.

This star has two-year-long eclipse that occurs every 27 years. But no one knows what eclipses the star, and the eclipse is very unusual. One of the possible models for epsilon Aurigae is that a large opaque disk seen nearly edge-on eclipses the primary star. The center of the disk might be partly transparent, due to the presence of one or more massive main-sequence stars. Because the disk is seen nearly edge-on to our line of sight, the supergiant star isn’t completely obscured even at the eclipse minimum.

Citizen Sky has put together materials guide you through the process of how to observe epsilon Aurigae, how to send in your observations, and then how to see your results, analyze them, and even publish them in a scientific journal! No previous experience is required. Citizen Sky hopes to involve thousands of people all over the world in real, active scientific research.

To learn more and get involved, visit Citizen Sky.

For more about epsilon Aurigae.

Posted on by Nancy Atkinson

Bouncing Boulders on Mars

Bouncing Boulder on Mars. Credit: NASA/JPL/U of A, Color and enhanement by Stuart Atkinson. Click for larger version.

[/caption]
Mars is not the dead world we once thought it was, and these images portray that fact. Stuart Atkinson (not a relative, but a good pal) is part of the UnmannedSpaceflight.com crew, the folks who have created fantastic visual treats from raw images sent back from our space exploring robots. Stu also has his own website, Cumbrian Sky . Today, Stu posted some pictures on his website, along with his story of how he came to create an utterly fantastic image from a rather ordinary-looking picture from the HiRISE camera on the Mars Reconnaisaince Orbiter. After seeing Stu’s handiwork today, I asked him if he wouldn’t mind sharing his story on Universe Today of how he came to make an amazing discovery on Mars. (Click on the image above for a larger version.)

HiRISE original image. Credit: NASA/JPL/U of AZ
HiRISE original image. Credit: NASA/JPL/U of AZ

Stu said he found the image above on the “dangerously addictive” Mars Global Data website. The image, of the Aram Chaos region, didn’t look very interesting at first. But then he zoomed in to get a better look at the “slumpy” feature in the middle of the image, and then zoomed in some more. “It looked like a section of the valley wall had come loose and slid down to the lower ground below,” Stu said as he raised a Spock-like eyebrow…
Zoom in of HiRISE original. Credit: Stuart Atkinson
Zoom in of HiRISE original. Credit: Stuart Atkinson

There, he discovered the track of a huge boulder that had “fallen from the valley wall and tumbled down, bouncing and rolling and boinging along for a long, long way.”

Adding color to the image really highlighted the tracks left by the bouncing boulder, and a few other tracks showed up as well, creating an absolutley awesome image and discovery!

“You know what I love about these images?,” Stu wrote on his blog. “They show movement, they show that things are moving on Mars right now. It’s not the dead, lifeless, corpse of a world I grew up with; it’s a world – we now know – where dust devils whirl and twirl across the plains… where clouds drift through the pale pink sky… and where huge stones fall from high cliff face, fall to the ground below, then bounce and roll and crash over it… unseen by anyone.”

“For now.”

Great detective work, Stu! I propose that this region should be named after Stuart Atkinson (at least the boulders, if nothing else!)

Also, check out Stu’s pictoral history of the International Space Station, starting from when it was a wee infant back in 1998 to the great images we’ve seen of the ISS this past week.

Posted on by Anne Minard

Calling All Amateur Astronomers: Help Comb Arecibo Data for Gems

[/caption]

Einstein@Home is one of the world’s largest public computing projects, with more than 200,000 people donating time on their own computers to mine gravitational wave data for the tell-tale signs of pulsars.

Now, Einstein@Home will begin searching Arecibo radio data to find binary systems consisting of the most extreme objects in the universe: a spinning neutron star orbiting another neutron star or a black hole. 

And the project needs even more public participation.

Today, Bruce Allen, director of the Einstein@Home project, and Jim Cordes, of Cornell University, announced that the Einstein@Home project is beginning to analyze data taken by the PALFA Consortium at the Arecibo Observatory in Puerto Rico. PALFA is the Pulsar Arecibo L-band Feed Array Consortium, an ongoing search effort. 

The Arecibo Observatory is the largest single-aperture radio telescope on the planet and is used for studies of pulsars, galaxies, solar system objects, and the Earth’s atmosphere. 

Current searches of radio data lose sensitivity for orbital periods shorter than about 50 minutes. But the enormous computational capabilities of the Einstein@Home project (equivalent to tens of thousands of computers) make it possible to detect pulsars in binary systems with orbital periods as short as 11 minutes.  The project is based at the University of Wisconsin in Milwaukee  and the Albert Einstein Institute in Germany.

“Discovery of a pulsar orbiting a neutron star or black hole, with a sub-hour orbital period, would provide tremendous opportunities to test General Relativity and to estimate how often such binaries merge,” said Cordes.

The mergers of such systems are among the rarest and most spectacular events in the universe. They emit bursts of gravitational waves that current detectors might be able to detect, and they are also thought to emit bursts of gamma rays just before the merged stars collapse to form a black hole. 

“While our long-term goal is to detect gravitational waves, in the shorter term we hope to discover at least a few new radio pulsars per year, which should be a lot of fun for Einstein@Home participants and should also be very interesting for astronomers,” Allen added. “We expect that most of the project’s participants will be eager to do both types of searches.”

Einstein@Home participants will automatically receive work for both the radio and gravitational-wave searches.

The large data sets from the Arecibo survey are archived and processed initially at Cornell and other PALFA institutions. For the Einstein@Home project, data are sent to the Albert Einstein Institute in Hannover via high-bandwidth Internet links, pre-processed and then distributed to computers around the world. The results are returned to AEI, Cornell, and UWM for further investigation.

You can join the Einstein@Home effort here.

LEAD IMAGE: Artist’s rendering of a neutron star. Credit: Space Telescope Science Institute.

Source: LIGO Scientific Collaboration Research Group