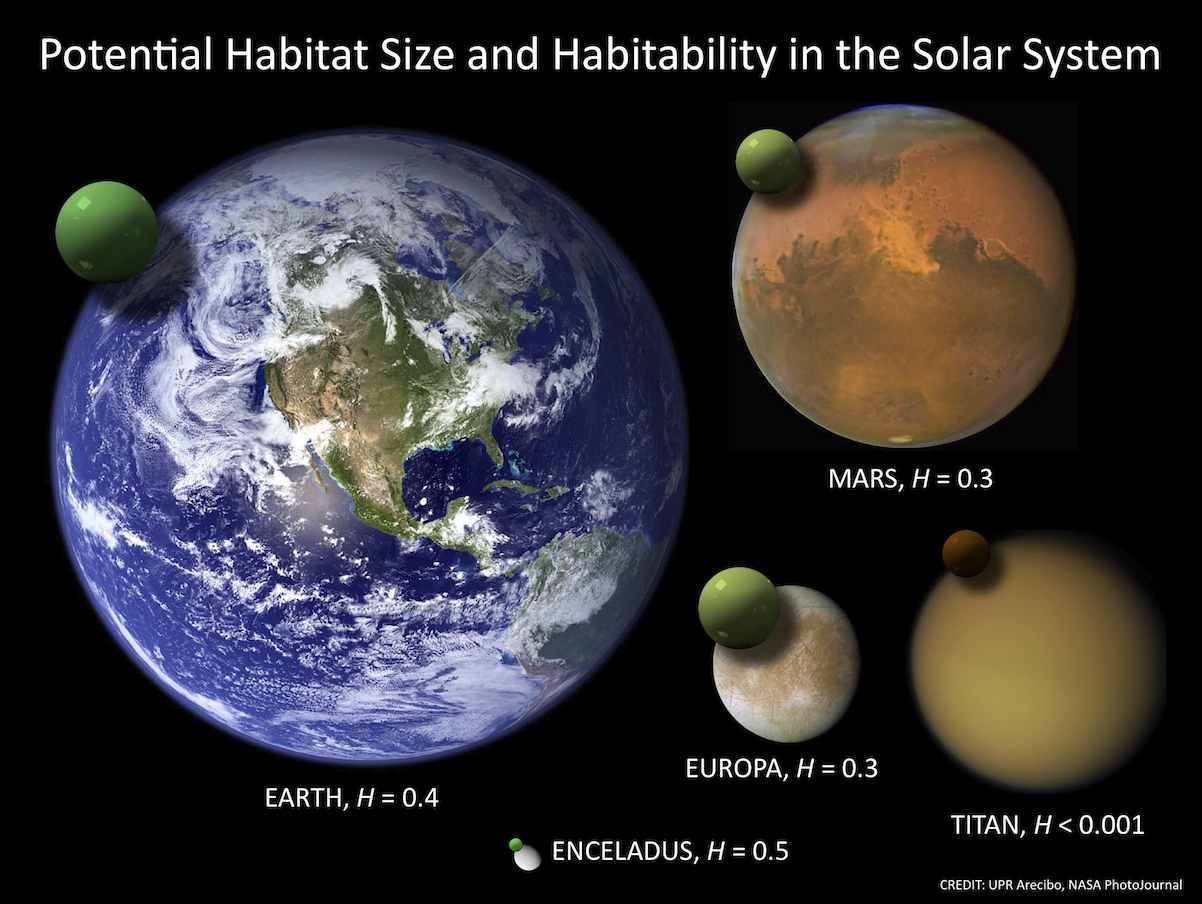
[/caption]
We live in a cosmic shooting gallery. In Phil Plait’s Death From the Skies, he lays out the dangers of a massive impact: destructive shockwaves, tsunamis, flash fires, atmospheric darkening…. The scenario isn’t pretty should a big one come our way. Fortunately, we may have a silent guardian: Jupiter.
Although many astronomers have assumed that Jupiter would likely sweep out dangerous interlopers (an important feat if we want life to gain a toehold), little work has been done to actually test the idea. To explore the hypothesis, a recent series of papers by J. Horner and B. W. Jones explores the effects of Jupiter’s gravitational pull on three different types of objects: main belt asteroids (which orbit between Mars and Jupiter), short period comets, and in their newest publication, submitted to the International Journal of Astrobiology, the Oort cloud comets (long period comets with the most distant part of their orbits far out in the solar system). In each paper, they simulated the primitive solar systems with the bodies in question with an Earth like planet, and gas giants of varying masses to determine the effect on the impact rate.
Somewhat surprisingly, for main belt asteroids, they determined, “that the notion that any ‘Jupiter’ would provide more shielding than no ‘Jupiter’ at all is incorrect.” Even without the simulation, the astronomers say that this should be expected and explain it by noting that, although Jupiter may shepherd some asteroids, it is also the main gravitational force perturbing their orbits and causing them to move into the inner solar system, where they may collide with Earth.
Contrary to the popular wisdom (which expected that the more massive the planet, the better it would shield us), there were notably fewer asteroids pushed into our line of sight for lower masses of the test Jupiter. Also surprisingly, they found that the most dangerous scenario was an instance in which the test Jupiter had 20% in which the planet “is massive enough to efficiently inject objects to Earth-crossing orbits.” However, they note that this 20% mass is dependent on how they chose to model the primordial asteroid belt and would likely change had they chosen a different model.
When the simulation was redone for for short period comets, they again found that, although Jupiter (and the other gas giants) may be effective at removing these dangerous objects, quite often they did so by sending them our way. As such, they again concluded that, as with asteroids, Jupiter’s gravitational jiggling was more dangerous than it was helpful.
Their most recent treatise explored Oort cloud objects. These objects are generally considered the largest potential threat since they normally reside so far out in the solar system’s gravitational well and thus, will have a greater distance to fall in and pick up momentum. From this situation, the researchers determined that the more massive the planet in Jupiter’s orbit, the better it does protect us from Oort cloud comets. The attribute this to the fact that these objects are initially so far from the Sun, that they are scarcely bound to the solar system. Even a little bit of extra momentum gained if they swing by Jupiter will likely be sufficient to eject them from the solar system all together, preventing them from settling into a closed orbit that would endanger the Earth every time it passed.
So whether or not Jupiter truly defends us or surreptitiously nudges danger our way depends on the type of object. For asteroids and short period comets, Jupiter’s gravitational agitation shoves more our direction, but for the ones that would potentially hurt is the most, the long period comets, Jupiter does provide some relief.